Recall we derived the Standard ‘ΛCDM’ Model of cosmology from Type-IIB SUGRA by identifying the inflaton with the Gukov-Vafa-Witten topologically hyper-twisted Kähler modulus with a Hořava-Witten uplift-embedding in M-theory. In this post, in the framework of Type-IIB ![]() system, I shall derive the two necessary conditions presupposed by such uplift, namely the de Sitter valley Coulomb gauge-phase and the Higgs waterfall gauge-phase that lead to the ground state Einstein-Sasaki-Minkowski vacuum. It is a remarkable feature of M-theory that it is the only quantum theory of gravity that can incorporate both phases. Let us recall some mathematical results I derived starting with the action:
system, I shall derive the two necessary conditions presupposed by such uplift, namely the de Sitter valley Coulomb gauge-phase and the Higgs waterfall gauge-phase that lead to the ground state Einstein-Sasaki-Minkowski vacuum. It is a remarkable feature of M-theory that it is the only quantum theory of gravity that can incorporate both phases. Let us recall some mathematical results I derived starting with the action:
![]()
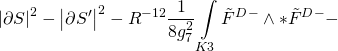
![Rendered by QuickLaTeX.com \displaystyle {{{{\left| {{{D}_{\mu }}\chi } \right|}}^{2}}-2{{g}^{2}}{{{\left| S \right|}}^{2}}{{{\left| \chi \right|}}^{2}}\left. {-\frac{{\left( {g_{3}^{2}+\tilde{g}_{3}^{2}} \right)}}{2}{{{\left( {{{\chi }^{\dagger }}{{\sigma }^{A}}\chi } \right)}}^{2}}} \right]}](https://www.georgeshiber.com/wp-content/ql-cache/quicklatex.com-b293d7bff887ed44402b6fe0144ca17d_l3.png)
Now take M-theory with parallel
branes spread along the orbifold
![]() , which preserves
, which preserves SUSY in 4-D, with the wrapped 6-D background along
![]() . Each M5 brane fills the 4-D non-compact spacetime and wraps the same holomorphic two-cycles
. Each M5 brane fills the 4-D non-compact spacetime and wraps the same holomorphic two-cycles ![]() on the Calabi-Yau manifold. The key terms of the 4-D
on the Calabi-Yau manifold. The key terms of the 4-D SYM theory are the volume modulus of the Calabi-Yau:

and the length modulus:
![]()
with the Type-IIB Calabi-Yau superpotential:
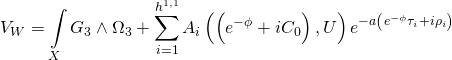
where the Ramond-Ramond gauge-coupling sector is given by the action:
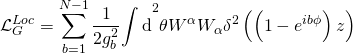
with the corresponding Chern-Simons action:
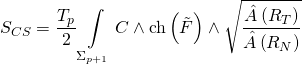
Via the F/M-theory duality, the M5 brane chiral superfields hence take the following form:
![]()
We then derived the P-term from the M2/M5 parallel brane system that supports the N 5-branes as such. The ![]() M2 Lagrangian takes the following form:
M2 Lagrangian takes the following form:
![]()
![]()
where ![]() is the covariant derivative:
is the covariant derivative:
![]()
![]() the Kähler potential, and the Chern-Simons term
the Kähler potential, and the Chern-Simons term ![]() for the gauge potential is given by:
for the gauge potential is given by:
![]()
![]()
and where ![]() define the brane transverse directions. The SUSY transformations are given by:
define the brane transverse directions. The SUSY transformations are given by:
![]()
![]()
![]()
with gauge conditions:
![]()
![]()
and with the Jacobi identity satisfied. Hence, we get the M5 brane Lagrangian by Nambu-Poisson deformations defined in terms of:
![]()
![]()
![]()
which promote the system to a 6D ![]() SYM system with a Lagrangian:
SYM system with a Lagrangian:
![]()
with:
![]()
![]()
and ![]() is given in terms of the kinetic terms for the
is given in terms of the kinetic terms for the ![]() ‘s:
‘s:
![]()
![]()
with:
![]()
and ![]() is given as such:
is given as such:
![]()
![]()
![]()
and where the relevant gauge field term is given as such:
![]()
with the Hodge dual field strength:
![]()
Hence, the equations of motion from ![]() are:
are:

Combining with the Bianchi identity:
![]()
yields:
![]()
The term for the B-field whose existence follows from the M2-Lagrangian, is:
![]()
giving us:
![]()
yielding solutions of the form:
![]()
Integrating, we get the ![]() terms, which, in our M2/M5 system, satisfy:
terms, which, in our M2/M5 system, satisfy:
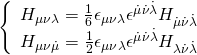

Plugging in the Kähler potential, we can derive the N=1 chiral super-field P-term action:
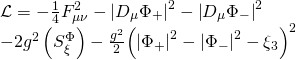
where we have:
![]()
and covariant derivative:
![]()
S is the neutral ![]() gauge field charge,
gauge field charge, ![]() is the N=2 hypermultiplet charged under
is the N=2 hypermultiplet charged under ![]() gauged by the N=2 vector multiplet
gauged by the N=2 vector multiplet ![]() with superpotential and D-term that drive inflation:
with superpotential and D-term that drive inflation:
![]()
![]()
dynamically as a function of kinetic terms of type ![]() . The proof proceeds by plugging the RG-flow equation with the Hubble and inflaton term factored quadratically, with the D-term potential:
. The proof proceeds by plugging the RG-flow equation with the Hubble and inflaton term factored quadratically, with the D-term potential:

Thus, the fermionic contributions to the inflaton field derive from the transformations:
![]()
![]()
![]()
and where the ![]() gravitino connection
gravitino connection ![]() is:
is:
![]()
and reduces to:
![]()
By integrating the N=1 chiral super-field P-term:
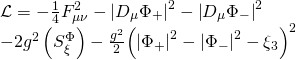
we get:
![]()
![]()
We can begin our embedding of hybrid inflation. First, note that a D3/D7 brane system in the presence of Fayet-Illiopoulos parameter becomes unstable unless it is a completely coincident system. Take the D7 brane world volume action:
![]()
where:
![]()
![]()
![]()
and where B is the pull-back of the NS-NS 2-form and ![]() is the Born-Infeld field strength. Now put the D7 brane in a D3 brane background. We get, for constant dilaton and metric:
is the Born-Infeld field strength. Now put the D7 brane in a D3 brane background. We get, for constant dilaton and metric:
![]()
and for the self-dual RR form:
![]()
where H is the central Hodge harmonic function on ![]() with
with ![]() the volume form on
the volume form on ![]() , while factoring in the D7 brane worldvolume gauge fields. Thus, our effective potential is given by:
, while factoring in the D7 brane worldvolume gauge fields. Thus, our effective potential is given by:
![]()
![]()
![]()
If the angles are equal, the force between the D3 brane and the D7 brane vanishes, giving us a 4-D Euclidean self-dual system in the 6 and 9 directions. In polar coordinates, we hence have:
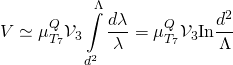
![]()
where ![]() is the renormalization group cutoff, and
is the renormalization group cutoff, and ![]() -symmetry allows us to deduce manifest supersymmetry breaking associated to the Yang-Mills field strength of the D3/D7 brane system. Our bosonic action is thus given by:
-symmetry allows us to deduce manifest supersymmetry breaking associated to the Yang-Mills field strength of the D3/D7 brane system. Our bosonic action is thus given by:
![]()
![]()
![]()
![]()
and some solutions must have some unbroken SUSY since there exists solutions to the kappa-symmetry equation:
![]()
where ![]() is the
is the ![]() -symmetry projection operator for a D7 brane in a D3 worldvolume background:
-symmetry projection operator for a D7 brane in a D3 worldvolume background:
![]()
![]()
and ![]() is a Type IIB spinor with a chiral bi-Majorana spinor representation, and
is a Type IIB spinor with a chiral bi-Majorana spinor representation, and ![]() is a Pauli matrix and in the absence of non-zero contorsion factor for a, the Killing equation reproduces the D7-brane projector:
is a Pauli matrix and in the absence of non-zero contorsion factor for a, the Killing equation reproduces the D7-brane projector:
![]()
corresponding to half of the unbroken supersymmetry. We hence have a skew-diagonal configuration with ![]() on the worldvolume, and the matrix
on the worldvolume, and the matrix ![]() is antisymmetric and independent of the worldvolume coordinates:
is antisymmetric and independent of the worldvolume coordinates:
![]()
![]()
with:
![]()
and the vielbeins are given by the D3-brane metric and the D3/D7 brane-system Killing spinors condition is:
![]()
![]()
The Killing spinor satisfies, in the presence of a D3 background, the following two conditions:
![]()
that break half the supersymmetry, and hence reductively yield:
![]()
![]()
![]()
The D3 brane worldvolume Hodge-Dirac harmonic function at the D7 loci ![]() is:
is:
![]()
Hence, the Killing equation has solution of type:

noting that in the Coulomb phase, unlike the Higgs phase, ![]() is a function of the D-brane worldvolume coordinates and thus determined by the RR-RR and NS-NS forms. Any such configuration of D3/D7 branes must be unstable. To see why, consider a D7 brane probed by a D3 brane with B field satisfying
is a function of the D-brane worldvolume coordinates and thus determined by the RR-RR and NS-NS forms. Any such configuration of D3/D7 branes must be unstable. To see why, consider a D7 brane probed by a D3 brane with B field satisfying ![]() . A SUSY solution deduced via mirror symmetry at the Hitchin holomorphic angles has the form:
. A SUSY solution deduced via mirror symmetry at the Hitchin holomorphic angles has the form:
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
Thus we have:
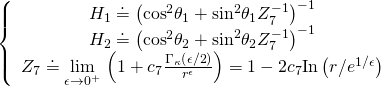
We then find that the D3 brane action is given by:
![]()
where we have implicitly defined ![]() by
by ![]() , and our potential is thus given by:
, and our potential is thus given by:
![]()
in light of the gauge-invariance of:
![]()
Now consider the D7 ![]() -symmetric Dirac-Born-Infeld/WZ action above, and a D3/D7 supersymmetric bound state for a given
-symmetric Dirac-Born-Infeld/WZ action above, and a D3/D7 supersymmetric bound state for a given ![]() and embed the D7 brane in the full Minkowski 10D space. The SUSY equation is then:
and embed the D7 brane in the full Minkowski 10D space. The SUSY equation is then:
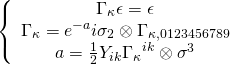
In the Coulomb hybrid phase, we pick an everywhere skew diagonal basis for ![]() , and in the Higgs phase, it can be allowed to be a function of the worldvolume coordinates. Hence
, and in the Higgs phase, it can be allowed to be a function of the worldvolume coordinates. Hence ![]() is a highly non-linear term given by:
is a highly non-linear term given by:
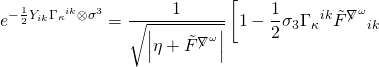
![]()
Thus, the Killing spinor equation reduces to:
![]()
![]()
with constant spinors. There are two ways to preserve SUSY. With an ![]() chiral/anti-chiral spinor satisfying the following conditions:
chiral/anti-chiral spinor satisfying the following conditions:
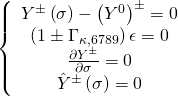
and with a spinor satisfying the equation:
![]()
![]()
Now, since the supersymmetric configurations in the Higgs branch are given by:
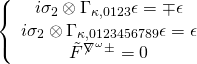
the solution necessarily has chiral spinors in Minkowski 4D spacetime and our system is equivalent to a ![]() -symmetric Euclideanized D3 brane dissolved into a D7 brane, as implied by the following relation:
-symmetric Euclideanized D3 brane dissolved into a D7 brane, as implied by the following relation:
![]()
Conjugation gives us the results in the Coulomb branch of hybrid inflation. We are now in a position to analyze a non-linear Seiberg-Witten solution to the above BPS equation. We put it in canonical Moriyama form:
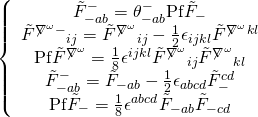
and our frame metric is defined implicitly via the open string metric:
![]()
and the vierbein and non-Abelian theta parameter are given by:
![]()
![]()
![]()
respectively, and the frame-Pfaffian equations are given by:
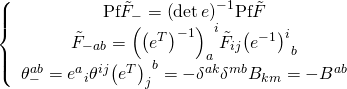
We can now derive the identity:
![]()
![]()
hence, our BPS equation reduces to:
![]()
![]()
To solve, note that ![]() can be defined in terms of the frame coordinates and the gauge potential as such:
can be defined in terms of the frame coordinates and the gauge potential as such:
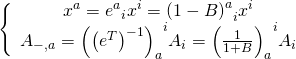
hence, a solution to:
![]()
has the following form:
![]()
with:
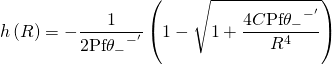
In the presence of the RR field, the ![]() -instanton gets a blow-up, and ceases to be singular and we get a UV non-linear Seiberg-Witten gauge equation:
-instanton gets a blow-up, and ceases to be singular and we get a UV non-linear Seiberg-Witten gauge equation:
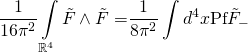
![]()
Thus, the non-vanishing ![]() is our cosmological potential seed that also defines a positive vacuum energy. Thus we get a hybrid slow-roll inflation stage where our pocket-universe goes through a waterfall condensation stage, and eventually settles into an Einstein-Sasaki-Minkowski vacuum described by a bound state of D3/D7 branes corresponding to the Higgs phase of the gauge theory with the FI term defined by
is our cosmological potential seed that also defines a positive vacuum energy. Thus we get a hybrid slow-roll inflation stage where our pocket-universe goes through a waterfall condensation stage, and eventually settles into an Einstein-Sasaki-Minkowski vacuum described by a bound state of D3/D7 branes corresponding to the Higgs phase of the gauge theory with the FI term defined by ![]() . Since D3 living on D7 branes can be interpreted as instantons due to Chern-Simons gauge coupling:
. Since D3 living on D7 branes can be interpreted as instantons due to Chern-Simons gauge coupling:
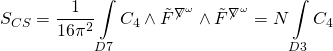
the Higgs phase is hence equivalent to a non-commutative Nekrasov-ADHM non-linear instanton in M-theory, and we have an intrinsic connection between the cosmological constant in 4D and the noncommutative ![]() parameter in internal space 6789. An uplift to M-theory is achieved via Kovalev twisted-connected-sum constructed
parameter in internal space 6789. An uplift to M-theory is achieved via Kovalev twisted-connected-sum constructed ![]() manifolds by gluing pairs of asymptotically cylindrical Calabi–Yau threefolds.
manifolds by gluing pairs of asymptotically cylindrical Calabi–Yau threefolds.