The String-String Duality, K3 Geometry, and Dimensional Reduction

By George Shiber Wednesday, November 23, 2016Saturday, August 17, 2019 In Cosmology, Grand Unification Physics, M Theory, Mathematical Physics, Mathematics, Philosophy Of Physics, Physics, Super String Theory, Supersymmetry Dimensional Reduction, K3 Geometry

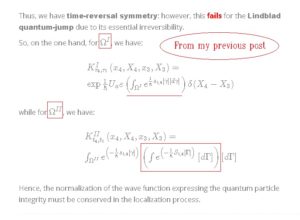
This post is on the String-String Duality. In particular, the D=6 string-string duality, which is crucial since it allows interchanging the roles of 4-D spacetime and string-world-sheet loop expansion, and this is mathematically essential for phenomenologically adequate string-compactifications. Here I will prove an equivalence between K3 membrane action and ${T^3} \times {S^1}/{Z^2}$ orbifold action and show how it entails D=6 string-string duality. Working in the bosonic sector, the membrane action is:

$\begin{array}{l}S = {S_M} + \int_{\partial {M^3}} {\left\{ {\frac{1}{2}} \right.} \left( {{g_{mn}}{\eta ^{ij}} + {b_{mn}}{\varepsilon ^{ij}}} \right)\\{{\not \partial }_i}{x^m}{{\not \partial }_j}{x^n} + \frac{1}{2}\left( {{g_{IJ}}{\eta ^{ij}} + {b_{IJ}}{\varepsilon ^{ij}}} \right)\\{{\not \partial }_i}{x^I}{{\not \partial }_j}{x^J} + {\varepsilon ^{ij}}{{\not \partial }_i}{x^J}{{\not \partial }_j}{x^m}\left. {A_m^J(x)} \right\}\end{array}$

where:

$\begin{array}{l}{S_M} = \int_{{M^3}} {\left( {\sqrt { - {g_{mn}}{{\not \partial }_i}{x^m}{{\not \partial }_j}{x^n}} } \right.} + \\\frac{1}{6}{\varepsilon ^{ijk}}{{\not \partial }_i}{x^m}{{\not \partial }_j}{x^n}{{\not \partial }_k}{x^p}\left. {{B_{mnp}}} \right)\end{array}$

Recall I derived, via Clifford algebraic symmetry, the total action:

$\begin{array}{l}{S^{Total}} = \frac{1}{{2\pi {\alpha ^\dagger }12}}\int\limits_{{\rm{world - volumes}}} {{d^{26}}} x\,d\,\Omega {\left( {{\phi _{INST}}} \right)^2}\sqrt {\frac{{ - {g_{\mu \nu }}}}{{ - \gamma }}} \,{e^{ - {c_{2n}}/{\Upsilon _\kappa }(\cos \varphi )}} \cdot \\\left( {{R_{icci}} - 4{{\left( {{{\not D}^{SuSy}}\left( {{\phi _{INST}}} \right)} \right)}^2}} \right) + \frac{1}{{12}}H_{3,\mu \nu \lambda }^bH_3^{b,\mu \nu \lambda }/A_\mu ^H + \sum\limits_{D - p - branes} {S_{Dp}^{WV}} \end{array}$

which is deep since Clifford algebras are a quantization of exterior algebras, and applying to the ‘Einstein-Minkowski’ tangent bundle, we get via Gaussian matrix elimination, an expansion of ${\not D^{SuSy}}$ via Green-functions, that yields M-Theory’s action:

${S_M} = \frac{1}{{{k^9}}}\int\limits_{{\rm{world - volumes}}} {{d^{11}}} \sqrt {\frac{{ - {g_{\mu \nu }}}}{{ - \gamma }}} {T_p}^{10}{\mkern 1mu} d{\mkern 1mu} \Omega {\left( {{\phi _{INST}}} \right)^{26}}\left( {{R_{icci}} - A_\mu ^H\frac{1}{{48}}G_4^2} \right) + \sum\limits_{Dp} {\not D_\mu ^{SuSy}} {e^{ - H_3^b}}/S_{Dp}^{WV} + \sum\limits_{Dp} {\not D_\nu ^{SuSy}} {e^{H_3^b}}/S_{Dp}^{SV}$

with $k$ the kappa symmetry term. With ${g_{mn}}$ the metric on ${M^{11}}$ , and ${x^m}$ the corresponding coordinates with ${B_{mnp}}$ an antisymmetric 3-tensor. The worldvolume ${M^3}$ is:

$R \times {S^1} \times {S^1}/{Z_2}$

The bosonic sector lives on the boundary of the open membrane: two copies of $R \times {S^1}$ , which naturally couple to the U(1) connections ${A^J}$ .

Now, double dimensional reduction of the twisted supermembrane on:

${M^{10}} \times {S^1}/{Z_2}$

of:

entails that the bosonic sector is that of the heterotic string:

$\begin{array}{l}{S_h}\int {{d^2}} \sigma \left\{ {\frac{1}{2}} \right.\left( {{g_{mn}}{\eta ^{ij}} + {b_{mn}}{\varepsilon ^{ij}}} \right){{\not \partial }_i}{x^m}{{\not \partial }_j}{x^n}\\ + \frac{1}{2}\left( {{g_{IJ}}{\eta ^{ij}} + {b_{IJ}}{\varepsilon ^{ij}}} \right){{\not \partial }_i}{x^I}{{\not \partial }_j}{x^I} + \\{\varepsilon ^{ij}}{{\not \partial }_i}{x^I}{{\not \partial }_n}{x^m}\left. {A_m^{(I)}(x)} \right\}\end{array}$

with gauge group indices I = 1, …, 16.

It gets interesting when we consider:

${M^{10}} = {T^3}{\rm{ }} \times {\rm{ }}{M^7}$

with dimension:

$dim{\rm{ }}{H^1}\left( {{M^7}} \right) = 0$

since the worldsheet action:

${S_{het}} = {S_{st}} + {S_{KK}} + {S_{\bmod }}$

is now just a sum of three terms:

${S_{st}} = \int {{d^2}} \sigma \frac{1}{2}\left( {{g_{mn}}{\eta ^{ij}} + {b_{mn}}{\varepsilon ^{ij}}} \right){\not \partial _i}{x^m}{\not \partial _j}{x^n}$

${S_{KK}}\int {{d^2}} \sigma {\varepsilon ^{ij}}{\not \partial _i}{x^I}{\not \partial _j}{x^m}A_m^I$

${S_{\bmod }} = \int {{d^2}} \sigma \frac{1}{2}\left( {{g_{IJ}}{\eta ^{ij}} + {b_{IJ}}{\varepsilon ^{ij}}} \right){\not \partial _i}{x^J}{\not \partial _j}{x^I}$

and the index I = 1, …, 22 labels 22 gauge fields: 16 coming from the internal dimensions of the heterotic string, and the other 6 gauge fields are the KK modes of the metric and antisymmetric tensor. The action ${S_{\bmod }}$ has a massless spectrum given by moduli fields corresponding to deformations of the Narain lattice and thus take values in the group manifold:

$\frac{{SO\left( {19,3} \right)}}{{SO\left( {19} \right) \times SO\left( 3 \right)}}$

Now, something fundamentally deep has occurred: all the gauge fields of the action 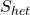${S_{het}}$ have appeared within a two-dimensional theory, and not a three-dimensional theory

This is precisely the long wavelength limit behavior of the open membrane:

the gauge fields are defined in terms of fields which live on 10-dimensional boundaries of M-theory

In the closed membrane case:

the gauge fields are defined in terms of 11-dimensional fields

Hence, the gauge fields of the closed membrane to be defined over M3 and not over its boundary, unlike the closed membrane, whose action on $K3 \times {M^7}$ is:

$\begin{array}{l}{{S'}_M} = \int_{{M^3}} {{d^3}} \zeta \left( {\sqrt { - {g_{mn}}{{\not \partial }_i}{x^m}{{\not \partial }_j}{x^n}} } \right.\\ + \frac{1}{6}{\varepsilon ^{ijk}}{{\not \partial }_i}{x^m}{{\not \partial }_j}{x^n}\left. {{{\not \partial }_k}{x^p}{B_{mnp}}} \right)\end{array}$

where ${M^3}$ is ${T^2} \times R$ with the spacetime being ${M^7} \times K3$ .

Hence, the closed membrane action ${S'_M}$ on ${M^7} \times K3$ reduces to:

${S'_M} = {S'_{st}} + {S'_{KK}} + {S'_{\bmod }}$

with:

${S'_{st}} = \int {{d^3}} \sigma \sqrt { - {g_{mn}}{{\not \partial }_i}{x^m}{{\not \partial }_j}{x^n}} + \frac{1}{6}{B_{mnp}}{\varepsilon ^{ijk}}{\not \partial _i}{x^m}{\not \partial _j}{x^n}{\not \partial _k}{x^p}$

${S'_{KK}} = \frac{1}{6}\int {{d^3}} \sigma {\varepsilon ^{ijk}}{\not \partial _i}{x^a}{\not \partial _j}{x^b}{\not \partial _k}{x^m}{B_{abm}}$

and

${S'_{\bmod }} = \int {{d^3}} \sigma \sqrt { - {g_{ab}}{{\not \partial }_i}{x^a}{{\not \partial }_j}{x^b}} + \frac{1}{6}{\varepsilon ^{ijk}}{\not \partial _i}{x^a}{\not \partial _j}{x^b}{\not \partial _k}{x^c}{B_{abc}}$

and since $K3$ surfaces have no one-cycles, it follows that the three-form potential that appears in ${S'_{KK}}$ of the action:

can be expanded in terms of the cocycles of $K3$ .

For the 22 2-cocycles of $K3$ , one can decompose $B$ in a similar way for the two-form potential:

${B_{abm}} = b_{ab}^I\left( {{x^a}} \right)C_m^I\left( {{x^r}} \right)$

with I = 1, …, 22 labeling the two-cycles of $K3$ . So after insertion into ${S'_{KK}}$ , we can derive:

$\int_{{M^3}} {{\varepsilon ^{ijk}}} {\not \partial _i}{x^m}{\not \partial _j}{x^b}{\not \partial _k}{x^a}b_{ab}^I\left( {{x^c}} \right)C_m^I\left( {{x^r}} \right)$

Applying reparametrization invariance, one can set:

$\rho = {x^{11}}$

where $\rho$ is a worldvolume coordinate, and now one performs a dimensional reduction of:

$\int_{{M^3}} {{\varepsilon ^{ijk}}} {\not \partial _i}{x^m}{\not \partial _j}{x^b}{\not \partial _k}{x^a}b_{ab}^I\left( {{x^c}} \right)C_m^I\left( {{x^r}} \right)$

Here are the key propositions relevant to the membrane/string duality of the low energy theory in D=7.

the kinetic terms for the gauge fields in D=7 supergravity are:

$\int_{{M^7}} {\sqrt { - {g^{\left( 7 \right)}}} } {a_{IJ}}F_{mn}^I{F^{Jmn}}$

derived by a split of the 4-4 field strength $H = dB$ , of the 11-dimensional supergravity action:

${H_{abmn}} = b_{ab}^IF_{mn}^I$

from the the following term:

$\begin{array}{l}\int_{{M^{11}}} {\sqrt { - {g^{\left( {11} \right)}}} } {H^2} = \int_{{M^7}} {\sqrt { - {g^{\left( 7 \right)}}} } F_{mn}^I{F^{Jmn}}\\\int_{K3} {\sqrt { - {g^{\left( {K3} \right)}}} } b_{ab}^I{b^{Jab}}\end{array}$

Membrane/string duality in D=7 requires the existence of a point in the moduli space of 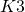$K3$ where all the 22 gauge fields are enhanced via U(1) gauging: this is key to preserving kappa symmetry. Thus, at the point in the moduli space when the 22 two-cycles vanish the following holds:

$\left\{ {\begin{array}{*{20}{c}}{{{\not \partial }_{{x^{11}}}}b_{ab}^I = 0}\\{{{\not \partial }_{{x^{11}}}}g_{ab}^I = 0}\end{array}} \right.$

Hence, dimensional reduction yields:

$\int_{{M^2}} {{\varepsilon ^{ij}}} {\not \partial _i}{x^m}{\not \partial _j}{x^b}b_{11b}^IC_m^I$

So, the S-duality map:

$\left\{ {\begin{array}{*{20}{c}}{b_{a11}^I{{\not \partial }_j}{x^a} \to {{\not \partial }_j}{x^I}}\\{C_m^I \to A_m^I}\end{array}} \right.$

takes:

$\int_{{M^2}} {{\varepsilon ^{ij}}} {\not \partial _i}{x^m}{\not \partial _j}{x^b}b_{11b}^IC_m^I$

to:

$\int_{{M^2}} {{\varepsilon ^{ij}}} {\not \partial _i}{x^I}A_m^I$

and is equivalent to the term ${S_{KK}}$ in:

${S_{\bmod }} = \int {{d^2}} \sigma \frac{1}{2}\left( {{g_{IJ}}{\eta ^{ij}} + {b_{IJ}}{\varepsilon ^{ij}}} \right){\not \partial _i}{x^J}{\not \partial _j}{x^I}$

So, the above map acts on the induced metric on the worldvolume: it follows then that the term in ${S'_{\bmod }}$ in:

yields, after a double dimensional reduction, of ${x^{11}}$ , the following:

$\int {{d^2}} \sigma \frac{1}{2}\left( {{g_{IJ}}{\eta ^{ij}} + {b_{IJ}}{\varepsilon ^{ij}}} \right){\not \partial _i}{x^J}{\not \partial _j}{x^I}$

with:

$\left\{ {\begin{array}{*{20}{c}}{{g_{IJ}} = {g_{ab}}b_I^{11a}b_J^{11b}}\\{{b_{IJ}} = {B_{ab11}}b_I^{11a}b_J^{11b}}\end{array}} \right.$

which yields an equivalence between:

$\int {{d^2}} \sigma \frac{1}{2}\left( {{g_{IJ}}{\eta ^{ij}} + {b_{IJ}}{\varepsilon ^{ij}}} \right){\not \partial _i}{x^J}{\not \partial _j}{x^I}$

and

${S_{\bmod }} = \int {{d^2}} \sigma \frac{1}{2}\left( {{g_{IJ}}{\eta ^{ij}} + {b_{IJ}}{\varepsilon ^{ij}}} \right){\not \partial _i}{x^J}{\not \partial _j}{x^I}$

Thus, the S-duality map that takes ${S_{KK}}$ to ${S'_{KK}}$ also takes ${S_{\bmod }}$ to dimensionally reduced ${S'_{\bmod }}$ .

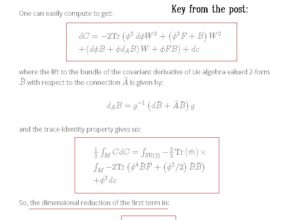
To achieve the matching of gauge sectors of the closed and open membrane, we must generate the gauge fields of the closed membrane before dimensionally reducing the theory, as opposed to the gauge fields of the open membrane, which are always generated within the two-dimensional theory. This explains the origin of strong-weak duality in string theory. The strong coupling limit of type IIA string is 11-dimensional supergravity which is believed to arise as the long wavelength limit of supermembrane theory. So, gauge fields present in the 3-dimensional theory will be strongly interacting, and will continue to be strongly interacting after dimensional reduction to a two-dimensional theory. However, the open membrane has its gauge fields appearing in two dimensional theories, which are therefore weakly interacting.

So, we must consider the spacetime part of the action for the closed membrane:

The term:

$\int_{{M^3}} {\sqrt { - {g_{mn}}{{\not \partial }_i}{x^m}{{\not \partial }_j}{x^n}} }$

can be dimensionally reduced to:

$\int_{{M^2}} {\sqrt { - {g_{mn}}{{\not \partial }_i}{x^m}{{\not \partial }_j}{x^n}} }$

which is equivalent to the first term in:

${S_{st}} = \int {{d^2}} \sigma \frac{1}{2}\left( {{g_{mn}}{\eta ^{ij}} + {b_{mn}}{\varepsilon ^{ij}}} \right){\not \partial _i}{x^m}{\not \partial _j}{x^n}$

and the term:

$\int_{{M^3}} {{\varepsilon ^{ijk}}} {\not \partial _i}{x^m}{\not \partial _j}{x^n}{\not \partial _k}{x^p}{B_{pmn}}$