The Cosmological Quantum State from Deformation Quantization

By George Shiber Saturday, December 24, 2016Friday, October 26, 2018 In Cosmology, Mathematical Physics, Mathematics, Philosophy, Philosophy Of Physics, Philosophy Of Science, Physics Deformation Quantization, Moyal-Wheeler-DeWitt equation

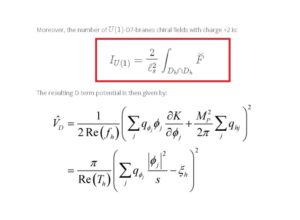
I will show that the Weyl-Wigner-Groenewold formalism of the cosmological quantum state is described by the Moyal-Wheeler-DeWitt deformation quantization equation with symplectic solutions in the Moyal-Wigner phase space: this is philosophically of foundational importance since quantum gravity necessitates that spacetime is quantized in a way described by the Moyal-Wheeler-DeWitt equation:

$\tilde H\left( {x + \frac{i}{2}{{\overrightarrow \partial }_x},{\Pi _x} - \frac{i}{2}{{\overrightarrow \partial }_x}} \right)W\left( {x,{\Pi _x}} \right) = 0$

where in the deformation quantization Hilbert space formalism, the deformed operator in the scalar product relative to $\tilde H$ is given by:

$\begin{array}{*{20}{l}}{\left\langle {\Psi ,{{\left( {d{X^\mu }d{X^\mu }} \right)}_\Theta }\Psi } \right\rangle = \left\langle {\Psi ,{{\left( {d{X^\mu }} \right)}^2}_\Theta \Psi } \right\rangle }\\{ = {{\left( {2\pi } \right)}^{ - d}}\mathop {\lim }\limits_{\varepsilon \to 0} \int {\int {dx{\mkern 1mu} dy{\mkern 1mu} {e^{ - ixy}}} } \chi \left( {\varepsilon x,\varepsilon y} \right) \cdot }\\{\left\langle {\Psi ,U(y)\alpha {\mkern 1mu} {\Theta _x}\left( {d{X^\mu }} \right)} \right\rangle }\\{ = {{\left( {2\pi } \right)}^{ - d}}\mathop {\lim }\limits_{\varepsilon \to 0} \int {\int {dx{\mkern 1mu} dy{\mkern 1mu} {e^{ - ixy}}} } \chi \left( {\varepsilon x,\varepsilon y} \right){b^\mu }\left( {x,y} \right)}\end{array}$

with $\Theta$ a skew-symmetric matrix on ${\mathbb{R}^d}$ , and $\chi \in f_{DQ}^{ps}\left( {{\mathbb{R}^d} \times {\mathbb{R}^d}} \right)$ , $\chi \left( {0,0} \right) = 1$ , and the deformation quantization differential operator is given by:

${\left( {d{X^\mu }} \right)^2}_\Theta = \int {{e^{ - 2{a_\mu }{{\left( {\Theta X} \right)}_\mu }}}} {\left( {d{X^\mu }} \right)^2}$

The central property of the cosmological quantum state is that it must entail the emergence of a classical universe satisfying all of the observable properties induced by the Friedmann-Robertson-Walker space-time, where such a FRW-space-time flat line-element, curved by quantum deformation, is:

${\left( {d{s^2}} \right)_\Theta } = d{\hat t^2} - {e^{H\hat t}}d{\hat x_ \bot }^2$

with curved space-time metric:

${\left( {{\eta _{\mu \nu }}} \right)_\Theta } = {e^{ - 2{a_\mu }{{\left( {\Theta \hat x} \right)}_\mu }}}{\eta _{\mu \nu }}$

derived from the 2-form tensored flat metric:

$\eta = {\eta _{\mu \nu }}d{\hat x^\mu }{ \otimes _{{{\bar A}_c}}}d{\hat x^\nu }$

where ${\bar A_c}$ is the Hilbert space representation algebra corresponding to the Schrödinger deformed differential structure, and the corresponding Minkowski metric is given by:

$d{s^2} = {\eta _{\mu \nu }}d{\hat x^\mu }d{\hat x^\nu }$

For an introduction to deformation quantization, this is a good read.

The hard part is that, due to the structure of FRW-minisuperspace, the quantization is not uniquely fixed up to isomorphism: there are infinite such transformations. A criterion of acceptability seems imposed on us, namely, that the state and quantization should probabilify the emergence of a classical limit in the topological neighborhood of classical FRW-predictions.

One must be careful in canonical quantization of Einsteinian gravity: the corresponding Hamiltonian is a linear combination of the FRW-constraints, and hence annihilates the physical quantum state, so we lose the time evolution from the theory. An equally grave problem arises if we try and apply canonical quantum gravity to cosmology, since the main mathematical entity is the wave function, thus obtained by Wheeler-DeWitt (WDW) equation or path integral, it must be possible to have an adequate wave packet that would peak around the classical FRW-cosmological model. Standardly, a wave-function collapse: reduction, in the Copenhagen interpretation would be adequate in quantum mechanics via a description of the dynamics of an ensemble of identical systems. In cosmology, there is only one Universe and hence only one system. Thus, the quantum state of the Universe has no well-defined wave-function. Add to that the fact that in quantum cosmology, the ‘observer‘ is an integral element of Universe. In any of the standard interpretations of quantum mechanics, a measurement involving a system, and ‘observer‘ [‘o’], and a measuring ‘device’ [‘m’], the quantum system interacts with a classical domain consisting, in part, of ‘o’, ‘m’, … , and it is this relational interaction that explains the reduction. However, quantum cosmology is a theory of the whole universe, and the measurement problem can have no standard quantum-mechanical solution, as can be attested by the fact that the cosmological quantum state ${\left| {{\psi _U}} \right\rangle _{\cos }}$ cannot satisfy the Lindblad collapse equation:

$\begin{array}{*{20}{l}}{d{\psi _t}^{S,m,o} = \left[ { - iHdt + \sum\limits_{k = 1}^n {\left( {{L_k} - {\ell _{k,t}}} \right)} } \right.}\\{d{W_{k,t}} - \frac{1}{2}\sum\limits_{k = 1}^n {\left. {\left( {L_k^\dagger {L_k} - 2{\ell _{k,t}}{L_k} + {{\left| {{\ell _{k,t}}} \right|}^2}} \right)} \right]} }\\{{\psi _t}^{S,m,o}}\end{array}$

since there can be no ‘m’, ‘o’ with Hilbert-separability with respect to ${\left| {{\psi _U}} \right\rangle _{\cos }}$ , given the unitarity of the Lindblad collapse operators ${\ell _{k,t}}$ :

${\ell _{k,t}} \equiv \frac{1}{2}\left\langle {{\psi _t},\left( {L_k^\dagger + {L_k}} \right){\psi _t}^{S,m,o}} \right\rangle$

as I showed: the Heisenberg equation with respect to the Lindblad collapse operators ${\ell _{k,t}}$ :

$\begin{array}{c}i\hbar \frac{{\rm{d}}}{{{\rm{d}}t}}{\ell _{k,t}}(t) = \left[ {{\ell _{k,t}}(t),\hat H(t)} \right]\\ + \frac{{\partial {\ell _{k,t}}(t)}}{{\partial t}}\end{array}$

has no solutions for ${\left| {{\psi _U}} \right\rangle _{\cos }}$ , as entailed by the Wheeler-DeWitt equation, and related, ${\left| {{\psi _U}} \right\rangle _{\cos }}$ satisfies the property of the Wheeler-DeWitt equation: namely, time totally disappears, and thus the above Lindblad collapse equation is actually undefinable for ${\left| {{\psi _U}} \right\rangle _{\cos }}$ , since ‘time‘ is essential to the Lindblad collapse operator ${\ell _{k,t}}$ .

This is the crucial role of deformation quantization: it transforms quantum cosmology into the Hamiltonian formalism of FRW-cosmology as it is a deformation of the structure of the algebra of observables and not a change in the characteristics or Hilbert-properties of the observables. 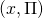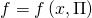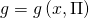In operator formalism, this is where the Weyl-Wigner-Groenewold-Moyal phase space quantization comes in, with a quantum system in terms of the classical c-number variables and Hilbert-space observables become classical functions of phase space $\left( {x,\Pi } \right)$ with the product $*$ for $f = f\left( {x,\Pi } \right)$ , $g = g\left( {x,\Pi } \right)$ , on a Poisson-Moyal manifold, is given by:

$f * g = \sum\limits_{n = 0}^\infty {{{\left( {i\hbar } \right)}^n}} {C_n}\left( {f,g} \right)$

with $\hbar$ the deformation quantization parameter and ${C_0}\left( {f,g} \right) = fg$ is the pointwise product, and the coefficients ${C_n}\left( {f,g} \right)$ are bi-differential operators with noncommutative product, satisfying the following conditions:

$\left\{ {\begin{array}{*{20}{c}}{{C_0}\left( {f,g} \right) = 0}\\{{C_1}\left( {f,g} \right) - {C_1}\left( {g,f} \right) = \left\{ {f,g} \right\}}\end{array}} \right.$

and

$\begin{array}{c}\sum\limits_{i + j = n} {{C_i}\left( {{C_j}\left( {f,g} \right),h} \right)} = \\\sum\limits_{i + j = n} {{C_i}\left( {f,{C_j}\left( {g,h} \right)} \right)} \end{array}$

that entail in the limit $\hbar \to 0$ , the star-product agrees with the pointwise products in classical phase space. Moreover, the commutation relation:

${\left[ {f,g} \right]_ * } = f * g - g * f$

converges to the Poisson bracket. In flat spaces, one defines a Moyal star product with Poisson tensor components as:

$\begin{array}{l}f\left( {x,\Pi } \right){ * _M}g\left( {x,\Pi } \right) = \\\exp \left( {\frac{{i\hbar }}{2}\left( {{{\overleftarrow \partial }_x}{{\overrightarrow \partial }_\Pi } - {{\overrightarrow \partial }_\Pi }{{\overleftarrow \partial }_x}} \right)} \right)g\left( {x,\Pi } \right)\\ = f\left( {x + \frac{{i\hbar }}{2}{{\overrightarrow \partial }_\Pi },\Pi - \frac{{i\hbar }}{2}{{\overrightarrow \partial }_\Pi }} \right)g\left( {x,\Pi } \right)\end{array}$

and from:

$f\left( {x + \frac{{i\hbar }}{2}{{\vec \partial }_\Pi },\Pi - \frac{{i\hbar }}{2}{{\vec \partial }_\Pi }} \right)g\left( {x,\Pi } \right)$

it follows that for a Moyal star product $A{ * _M}B$ of any two functions $A$ and $B$ , the Weyl-Wigner correspondence is:

$\begin{array}{l}\left( {A{ * _M}B} \right)\left( {x,\Pi } \right) = \\A\left( {x + \frac{{i\hbar }}{2}{\partial _\Pi },\Pi - \frac{{i\hbar }}{2}{\partial _x}} \right)B\left( {x,\Pi } \right)\end{array}$

where the operator:

$A\left( {x + \frac{{i\hbar }}{2}{\partial _\Pi },\Pi - \frac{{i\hbar }}{2}{\partial _x}} \right)$

acts on the phase space ${\tilde C^\infty }$ .

Hence, we can replace the position and momentum variables in $A$ by the Bopp-pseudo-operators containing position and momentum and their derivatives:

$\left\{ {\begin{array}{*{20}{c}}{x \to x + \frac{{i\hbar }}{2}{\partial _\Pi }}\\{\Pi \to \Pi - \frac{{i\hbar }}{2}{\partial _x}}\end{array}} \right.$

and they act on functions or distributions defined on the noncommutative phase space ${\mathbb{R}^D} \oplus {\mathbb{R}^D}$ rather than a Weyl-action on functions defined on ${\mathbb{R}^D}$ . The Bopp-pseudo-differential operators are the basis on deformation quantization that essentially reduces to a Weyl tensor calculus. Moreover, the following equation:

entails that noncommutative quantum mechanics reduces to a Bopp operator tensor calculus.

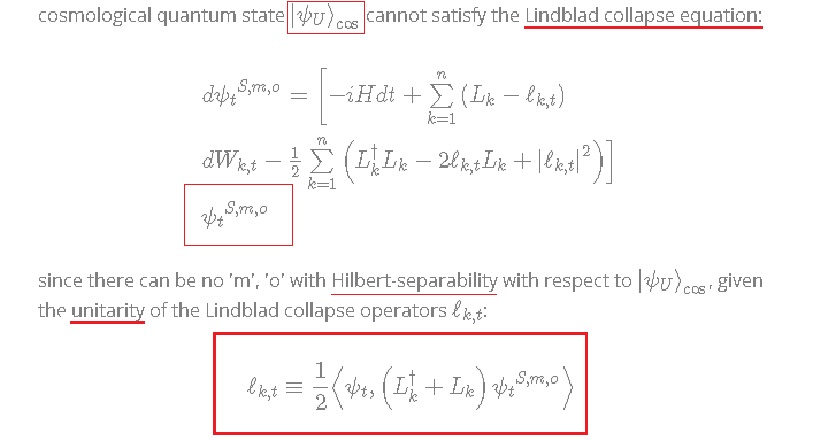
The central notion in deformation quantization is the Wigner quasiprobability distribution function, visualizable as:

with quantum Schrödinger-cat spectroscopy states that can be visualized as:

Thus, as can be seen, it is a generating function for all spatial auto-correlation functions of a given quantum mechanical wave-function, and the Wigner function with wave-function of system ${\psi _n}(x)$ in a $2D$ -dimensional phase space is:

${W_n}\left( {x,\Pi } \right) = \frac{1}{{{{\left( {2\pi \hbar } \right)}^D}}}\int {\psi _n^ * } \left( {x - \frac{\hbar }{2}y} \right){e^{ - i\frac{{\Pi \cdot y}}{\hbar }}}{d^D}y$

the crucial product term being:

${e^{ - i\frac{{\Pi \cdot y}}{\hbar }}}{d^D}y$

thus, quantum mechanical expectation-values of observables and transition amplitudes are phase space integrals of c-number functions, weighted by the Wigner function

Let us delve now into an analysis of quantum cosmology of a flat Friedmann-Lemaître-Robertson-Walker (FLRW) universe with radiative dust or cosmic strings.

There are two foundationally different ways of quantizing Einstein’s TGR: the canonical quantum gravity programme and the ‘particle-physics’-paradigmatic one, where the graviton is the quanta of the gravitational field and it propagates in a background Minkowski spacetime, and like all elementary particles, is associated with a specific representation of the Poincaré group and the background becomes a Minkowski spacetime after a suitable fixing of the background topology and differential structure of Riemannian spacetime manifold. Then one splits it into a background ${\eta _{\mu \nu }}$ part, dynamical ${h_{\mu \nu }}$ part, and the field ${h_{\mu \nu }}$ is then quantized by standard field-theoretic methods given the axiom that the gravitational interaction involves the exchange of gravitons. The canonical approach starts with a foliation of spacetime with respect to which the canonical variables are defined. Thus, the quantization of gravity is a quantization of the metrical structure of spacetime directly, satisfying a dynamical principle and dynamical equations of geometrodynamics. In such an approach, the difficulties are best appreciated by analyzing the minisuperspace method: basically, one picks a minisuperspace of definite symmetry and we hypothesize that the symmetry is preserved under the quantization process, and in order to preserve a definite symmetry for the minisuperspace, we require matter-sources: a perfect fluid, Yang-Mills fields, scalar fields or spinor fields, that are also quantized. However, at the Planck scale, the domain of quantum geometrodynamics gives matter-sources that differ from that of quantum field theory, hence, in quantum geometrodynamics, the source(s) is (are) taken factored only formally by the addition of new degrees of freedom to the equations that describe the dynamical geometry. Due to canonicality, general exact solutions cannot be found in the presence of Yang-Mills, scalar or spinor fields, and the Hilbert space structure is ill-defined and there is no way to recover semiclassical time. Moreover, the Bekenstein-Bound implies that the information content of the very early Universe is zero and the only physical variables one can take into account are the scale factor of the Universe, the density and pressure of the matter field. So we must quantize the FLRW universe for a perfect fluid. The advantage though, is that a perfect fluid introduces a variable that can naturally be identified with time, hence leading to a well-defined Hilbert space structure. Another advantage is that the degrees of freedom allow us to treat the barotropic equation of state, which yields general exact solutions. Thus, the line element of the spatially flat FLRW universe is given by:

$d{s^2} = - {N^2}\left( t \right)d{t^2} + {a^2}\left( t \right)\left( {d{x^2} + d{y^2} + d{z^2}} \right)$

where $N\left( t \right)$ is the lapse-function and $a\left( t \right)$ is the scale factor. The action functional consisting of the gravitational and the matter part satisfying a perfect fluid dynamics is then:

$S = \frac{1}{{16\pi G}}\int R \sqrt { - g} {d^4}x - \int \rho \sqrt { - g} {d^4}x$

with $g$ the determinant of the spacetime metric, $R$ the Ricci scalar, and $\rho = \sum\limits_i {{\rho _i}}$ is the total energy density satisfying:

${\rho _i} = {\rho _{0i}}{\left( {\frac{a}{{{a_0}}}} \right)^{ - 3\left( {{\omega _i} + 1} \right)}}$

where ${\omega _i}$ is the equation-of-state parameter of the $i$ -th component of matter-fluid and ${\rho _{i0}}$ is the energy density at the measuring epoch. Hence:

$S = \frac{1}{{16\pi G}}\int R \sqrt { - g} {d^4}x - \int \rho \sqrt { - g} {d^4}x$

reduces to:

$\begin{array}{l}S = - \frac{{3{V_3}}}{{8\pi G}}\int {N{a^3}} \left( {\frac{a}{{{N^2}}}} \right.{\left( {\frac{{da}}{{dt}}} \right)^2} + \\\frac{{8\pi G}}{3}\sum\limits_i {{\rho _{i0}}\left. {{{\left( {\frac{a}{{{a_0}}}} \right)}^{ - 3\left( {{\omega _i} + 1} \right)}}} \right)} dt\end{array}$

with:

${V_3} = \int {{d^3}} x$

the spatial volume 3-metric. Take now the Hilbert-space measurable quantities of FLRW cosmology. We define a cosmological lapse-function $\tilde N = \frac{N}{x}$ and rescale the energy density in terms of the corresponding density parameters

${\Omega _i} = \frac{{8\pi G{\rho _{0i}}}}{{3H_0^2}}$

with ${H_0}$ the Hubble parameter, and so we have the following relation:

${\rho _i} = \frac{{3H_0^2{\Omega _i}}}{{8\pi G}}{\left( {\frac{a}{{{a_0}}}} \right)^{ - 3\left( {1 + \,{\omega _i}} \right)}}$

So, a dimensionless scale factor $x = \frac{a}{{{a_0}}}$ is induced as well as a new dimensionless time coordinate $\eta = {H_0}t$ . Then the Lagrangian of our model in the conformal frame up to a multiplicative constant

$\frac{{3{V_3}a_0^3{H_0}}}{{4\pi G}}$

is:

$L = - \frac{1}{2}\left( {\frac{{{{\dot x}^2}}}{{\tilde N}} + \tilde N\sum\limits_i {{\Omega _i}{x^{1 - 3{\omega _i}}}} } \right)$

and $\dot x$ is differentiation respect to $\eta$ , and the conjugate momentum and the primary constraint are given by:

${{\Pi _x} = \frac{{\partial L}}{{\partial \dot x}} = - \frac{{\dot x}}{{\tilde N}}}$

and

${\Pi _{\tilde N}} = \frac{{\partial L}}{{\partial \frac{{d\tilde N}}{{d\eta }}}} = 0$

So the corresponding Hamiltonian will be:

$H = {\tilde N_l}\left( { - \frac{{{\Pi _x}^2}}{2} + \frac{1}{2}\sum\limits_i {{\Omega _i}{x^{1 - 3{\omega _i}}}} } \right)$

where ${\tilde N_l}$ is the Lagrange multiplier, which enforces the following Hamiltonian constraint:

${\tilde H_s} = - \frac{{{\Pi _x}^2}}{2} + \frac{1}{2}\sum\limits_i {{\Omega _i}} {x^{1 - 3{\omega _i}}} = 0$

where ${\tilde H_s}$ is the super-Hamiltonian, and at the initial time ${\eta _0} = {t_0}{H_0}$ , reduces to well-known relation between the density parameters $\sum\limits_i {{\Omega _i}} = 1$ .

The deformation quantization is carried out by replacing the ordinary product of the observables in phase-space by the Moyal product. Thus, the Hamiltonian condition:

${\tilde H_s} = - \frac{{{\Pi _x}^2}}{2} + \frac{1}{2}\sum\limits_i {{\Omega _i}} {x^{1 - 3{\omega _i}}} = 0$

yields the Moyal-Wheeler-DeWitt equation:

$\tilde H\left( {x + \frac{i}{2}{{\overrightarrow \partial }_x},{\Pi _x} - \frac{i}{2}{{\overrightarrow \partial }_x}} \right)W\left( {x,{\Pi _x}} \right) = 0$

By substituting the above Hamiltonian condition by its deformed version:

$\tilde H\left( {x,{\Pi _x}} \right){ * _M}{W_n}\left( {x,{\Pi _x}} \right) = 0$

and the fact that the product ${ * _M}$ involves exponentials of derivative operators implies that the Moyal-Wheeler-DeWitt equation is equivalent to:

$\tilde H\left( {x + \frac{i}{2}{{\overrightarrow \partial }_{{\Pi _ * }}},{\Pi _x} - \frac{i}{2}{{\overrightarrow \partial }_x}} \right)W\left( {x,{\Pi _x}} \right) = 0$

Take the classical-quantum correlation in a radiation-dust filled universe. Then the classic super-Hamiltonian reduces to:

$\Pi _x^2 - {\Omega _d}x - {\Omega _r} = 0$

with ${\Omega _d}$ and ${\Omega _r}$ the density parameters of dust and radiation, satisfying:

${\Omega _d} + {\Omega _r} = 1$

at initial cosmic epoch ${\eta _0}$ . Moreover, the Moyal-Wheeler-DeWitt equation reduces to:

$\left( {{{\left( {{\Pi _x} - \frac{i}{2}{{\overrightarrow \partial }_x}} \right)}^2} - {\Omega _d}\left( {x - \frac{i}{2}{{\overrightarrow \partial }_{{\Pi _x}}}} \right) - {\Omega _r}} \right)W = 0$

with an ordering of the kinetic term:

$\begin{array}{c}\Pi _x^2 \to {x^{ - \alpha }} * {\Pi _x} * {x^\alpha } * {\Pi _x}\\ = \Pi _x^2 - i{\alpha ^{ - 1}} * {\Pi _x}\end{array}$

where $\alpha$ factors the ordering ambiguity.

Without any loss of generality, I will work with the Laplace-Beltrami operator of the conformal frame of the corresponding Wheeler-DeWitt equation, thus, $\alpha = 0$ . Hence, the Wigner function is real-valued, so by separation of the real and imaginary parts of the Moyal-Wheeler-DeWitt equation, we get two coupled partial differential equations:

$\left( { - \frac{1}{4}\partial _x^2 - {\Omega _d}x + \Pi _x^2 - {\Omega _r}} \right)W\left( {x,{\Pi _x}} \right) = 0$

and

$\left( {{\Pi _x}{\partial _x} + \frac{{{\Omega _d}}}{2}{\partial _{{\Pi _x}}}} \right)W\left( {x,{\Pi _x}} \right) = 0$

where the first equation is the partial derivatives of ${\Pi _x}$ , and the second equation is a phase-space equation that enforces a special symmetry on the solutions.

Now, the solution to:

$\left( {{\Pi _x}{\partial _x} + \frac{{{\Omega _d}}}{2}{\partial _{{\Pi _x}}}} \right)W\left( {x,{\Pi _x}} \right) = 0$

is given by:

$W = f\left( {\Pi _x^2 - {\Omega _d}x} \right)$

with $f$ any real function.

Now define a new variable

$\zeta = \Pi _x^2 - {\Omega _d}x$

hence, the following relation:

$\frac{{{\partial ^2}W\left( {x,{\Pi _x}} \right)}}{{{\partial _x}^2}} = \Omega _d^2\frac{{{d^2}f\left( \zeta \right)}}{{d{\zeta ^2}}}$

follows from the chain rule. Thus, the first partial differential equation:

$\left( { - \frac{1}{4}\partial _x^2 - {\Omega _d}x + \Pi _x^2 - {\Omega _r}} \right)W\left( {x,{\Pi _x}} \right) = 0$

reduces to the second order ordinary differential equation:

$- \frac{{\Omega _d^2}}{4}\frac{{{d^2}f\left( \zeta \right)}}{{d{\zeta ^2}}} + \left( {\zeta - {\Omega _r}} \right)f\left( \zeta \right) = 0$

whose finite value solution is:

$W\left( {x,{\Pi _x}} \right) = {N'_ \bot }{A_i}\left( {{{\left( {\frac{2}{{{\Omega _d}}}} \right)}^{2/3}}\left( {\Pi _x^2 - {\Omega _d}x - {\Omega _r}} \right)} \right)$

with $Ai\left( \xi \right)$ the Airy function of first kind and

${N'_ \bot } = \frac{1}{{2\pi }}{\left( {\frac{2}{{{\Omega _d}}}} \right)^{2/3}}$

Then the extremum loci of the Wigner function is the deformed super-Hamiltonian:

$\Pi _x^2 - {\Omega _d}x - {\Omega _r} + {\left( {\frac{{{\Omega _d}}}{2}} \right)^{2/3}}{a_n} = 0$

and the ${a_n}$ ‘s are the zeroes of derivative Airy functions:

$\frac{d}{{d\xi }}Ai\left( { - \xi } \right)\left| {_{\xi = {a_n}}} \right.$

and the negatives of $Ai$ , for n = 1, 2, 3, have solutions-values as:

$\left\{ {\begin{array}{*{20}{c}}{1:{a_n}\quad 1.0187...}\\{2:{a_n}\quad 3.2481...}\\{3:{a_n}\quad 4.8200...}\end{array}} \right.$

and coincide with the classical solution:

$\Pi _x^2 - {\Omega _d}x - {\Omega _r} = 0$

with the deformation valued modification of the density parameter of radiation given by:

${\tilde \Omega _r}\left( n \right) = {\Omega _r} - {\left( {\frac{{{\Omega _d}}}{2}} \right)^{2/3}}{a_n}$

Therefore, we get the right result – all solutions are non-singular iff:

${\Omega _r}{\left( {\frac{{{\Omega _d}}}{2}} \right)^{2/3}} < {a_1}$

Let us now turn to the classical-quantum correlation of a universe filled with a cosmic string perfect fluid, and with 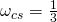${\omega _{cs}} = \frac{1}{3}$ . Then the classical super-Hamiltonian condition above is given by:

$\Pi _x^2 - {\Omega _{cs}}{x^2} = 0$

and the Moyal-Wheeler-DeWitt equation reduces to:

$\left( {{{\left( {{\Pi _x} - \frac{i}{2}{{\overrightarrow \partial }_x}} \right)}^2} - {\Omega _{cs}}{{\left( {x + \frac{i}{2}{{\overrightarrow \partial }_{{\Pi _x}}}} \right)}^2}} \right)W = 0$

Separating the real and imaginary parts gives us two independent equations:

$\left( {{\Omega _{cs}}x{\partial _{{\Pi _x}}} + {\Pi _x}{\partial _x}} \right)W = 0$

and

$\left( {\Pi _x^2 - {\Omega _{cs}}{x^2} - \frac{1}{4}\partial _x^2 + \frac{{{\Omega _{cs}}}}{4}\partial _{{\Pi _x}}^2} \right)W = 0$

The classical singularity finite-value solution is given by:

$W\left( {x,{\Pi _x}} \right) = \frac{1}{{2\pi \sqrt {{\Omega _{cs}}} }}{J_0}\left( {\frac{{{\Pi _x}^2 - {\Omega _{cs}}{x^2}}}{{\sqrt {{\Omega _{cs}}} }}} \right)$

${J_0}\left( \xi \right)$ being the zero-order Bessel function. So the extremum loci of the Wigner function are:

$\Pi _x^2 - {\Omega _{cs}}{x^2} - \sqrt {{\Omega _{cs}}} {j_n} = 0$

with ${j_n}$ the zeroes of derivative Bessel function, and we get the modified table:

$\left\{ {\begin{array}{*{20}{c}}{1:{j_n}\quad 0}\\{2:{j_n}\quad 3.831...}\\{3:{j_n}\quad 7.015...}\end{array}} \right.$

and the density parameter of cosmic radiation is given by:

${\Omega _r} = \sqrt {{\Omega _{cs}}} {j_n}$

And the key is, the above extremum loci of the Wigner function table is completely quantum in origin and the corresponding classical universe is filled only with the cosmic string fluid. At later times, where the universe is cosmic string dominated, both models are isomorphic. Yet for very small values of the scale factor ${\hat x_ \bot }$ , the emergent universes for various values of ${j_n}$ are non-singular and radiation dominated. The first zero of derivative Bessel function is zero, thus for ${j_0} = 0$ , quantum cosmology predicts a cosmic string filled singular universe. For large values of the scale factor ${\hat x_ \bot }$ , there is a perfect correlation between the quantum quasi-probability distribution and the classical trajectory in phase space!