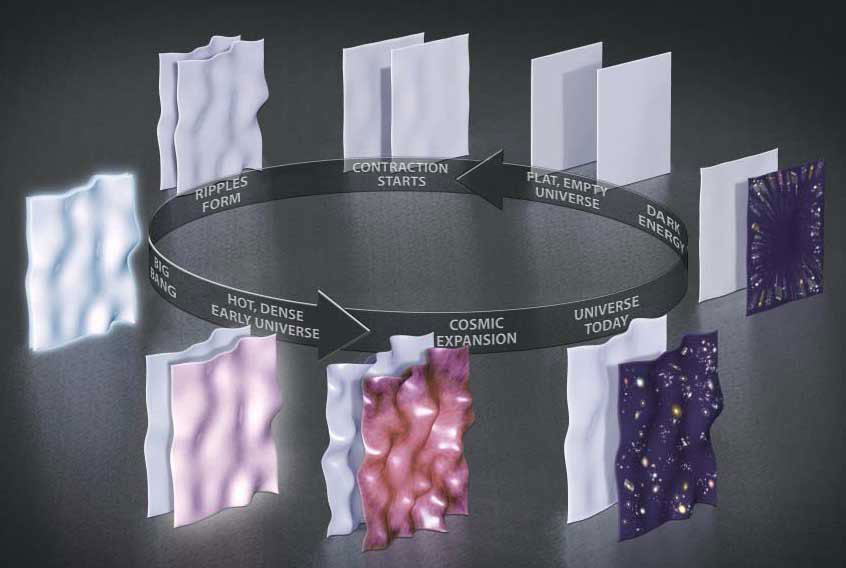
SuSy, Dp-Branes, And Green-Schwarz Analysis: The Sparticle Vacua And The Big Bang

By George Shiber Monday, June 29, 2015Friday, October 26, 2018 In Grand Unification Physics, M Theory, Mathematical Physics, Philosophy Of Mathematics, Philosophy Of Physics, Philosophy Of Science, Super String Theory, Supersymmetry Big Bang, bosons

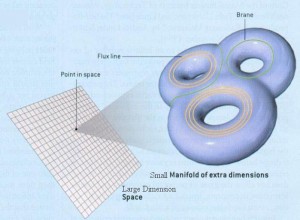
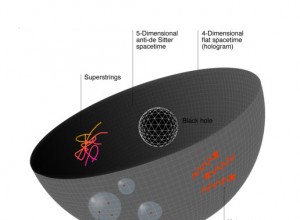
One may wonder, What came before the Big Bang? If space-time did not exist then, how could everything appear from nothing? . . . Explaining this initial singularity —where and when it all began—still remains the most intractable problem of modern cosmology, science and philosophy in general. ~ Andrei Linde: I might add – will always remain so, never to be ‘solved’!

An equation means nothing to me unless it expresses a thought of God. S. Ramanujan!

In my last post, I showed that the kappa symmetric algebraic equation:

${\Gamma _\kappa }\left| {_{{\rm{Bo}}{{\rm{s}}^\varepsilon }}} \right. = \varepsilon$

has a Sasaki-Einstein solution. It can be seen that any such solution generates the SUSY vacuum for the sparticle spectrum:

${\not {\rm Z}_V} = {T_{M_L^{p + 1}}}dy \wedge dz = {T_{{M^{p + n}}}}\frac{1}{{2\pi ik}}\int_{M_L^{p + 1}} {d\,\Omega } {({\phi _{si}})^{p + n}} \wedge d\;\widetilde \Omega {({\phi _{si}})^{ - p + 1}}$

with ${\phi _{si}}$ being the string variable on the corresponding 2-D world-sheet, and $M$ the super-Lagrangian manifold, and solving gives us a exact and complete description of the sparticle fields ‘living’ on:

$Ad{S_5} \times E_S^5$

with $E_S^5$ being a 5-D Sasaki-Einstein manifold. Let me now, in this context, raise deep philosophical problems, for both, ${\not {\rm Z}_V}$ : which is critical for explaining Higgs physics, and for the Big Bang Theory. Note first, that by $e = m{c^2}$ and the Heisenberg uncertainty principle for time and energy, denoted here by ${H_{E,t}}$ , the expression in ${\not {\rm Z}_V}$ , namely:

$\int_{M_L^{p + n}} {d\,\Omega } {({\phi _{si}})^{p + n}}$

can be diffeomorphically transformed into a derivative funtor of the string variable as a function of time: ${\phi _{si}}(t)$ , and thus, we get:

$\frac{d}{{d{\sigma _t}}}\int_{M_L^{p + n}} {d\,\Omega } ({\phi _{si}}^{p + n}(t))$

Now, by ${H_{E,t}}$ , given that the Dp+n brane tension ${T_{Dp + n}}$ , by unitarity, always has positive-definite energy, ‘time‘ is hence in quantum superposition with respect to energy, thus

$\frac{d}{{d{\sigma _t}}}\int_{M_L^{p + n}} {d\,\Omega } ({\phi _{si}}^{p + n}(t))$

will have no solution, entailing that the super-Lagrangian manifold $M_L^{p + n}$ is degenerate topologically and can neither admit a Ricci tensor nor a Minkowskian metric: hence, ${\not {\rm Z}_V}$ cannot be the Kähler vacua that generates the sparticles, and since the existence of the Higgs superpartner is a necessary condition for a complete explanation of the Gibbs-cosmological mass distribution, we have a catastrophe, since that implies the universe has no matter, and that really matters! Moreover, and more severe, is that ‘time‘ as it occurs in the string variable, converges to zero, in the initial Dp+n singularity integral, at Big Bang ‘time’:

${\not \Delta ^D}\varsigma = \underbrace {\int_0^{\delta {f_K}} {\frac{{d{t^ \circ }}}{{a({t^ \circ })}}\not D{{\not {\rm Z}}_V}} }_{{\rm{Quantum Gravity}}} + \underbrace {\int_{\delta f}^{{f_K}} {\frac{{d{t^ \circ }}}{{a({t^ \circ })}}d\,\Omega {{({\phi _{si}})}^{e{\phi _{si}}({t^ \circ })}}d{\Phi _I}} }_{{\rm{Inflation}}}$

where ${f_K}$ is the Yukawa interactional entropic measure of curvature ‘near’ the singularity, and $a(t)$ describes the inflationary expansion, with:

$d\,\Omega = d{\theta ^2} + \sin d\Phi _I^2$

with ${\Phi _I}$ the instanton scalar field and $K$ the Guassian curvature. So, by ${H_{E,t}}$ :

$\frac{d}{{d{\sigma _t}}}\underbrace {\int_0^{\delta {f_K}} {\frac{{d{t^ \circ }}}{{a({t^ \circ })}}\not D{{\not {\rm Z}}_V}} }_{{\rm{Quantum Gravity}}}d({\phi _{si}}({t^ \circ })$

has four solutions: one describing the expansion of space in positive-time direction, one in negative, and one in both (the Robinson solution) and one in none. The last 2 solutions are clearly incoherent since that would imply not only that the Big Bang did not ‘occur’ by the right-hand-side of ${\not \Delta ^D}\varsigma$ , but also by $e = m{c^2}$ applied to ${\not {\rm Z}_V}$ , space not only could not have ‘started’ to expand, but actually we get an anti-‘Big-Bang’: space started, at initial time = 0, to contract into the ‘past’! The only way to solve the above-mentioned problems in a way that is consistent with any theory of quantum gravity is solving the kappa symmteric p-brane Green-Schwarz action with chiral anomaly cancellation. Let us consider a p-brane in a coset $G/H$ with $G$ a super-Poincaré group, $H$ the corresponding Lorentz proper subgroup. A parametrization can be expressed as:

$g(z) = {e^{i({x^a}{P_a} + \widetilde \theta Q)}}$

and the vielbein on $G/H$ can be derived via the Cartan form:

$g{(z)^{ - 1}}dg(z) = i{\prod ^a}{P_a} + id\widetilde \theta Q\,\not \partial \phi _{si}^{ - 1} = i\left( {d{x^a} - i\left( {\widetilde \theta {\Gamma ^a}d\theta \,\not \partial \phi _{si}^{ + 1}} \right)} \right){P_a} + id\widetilde \theta Q$

which is invariant under SySy transformations:

${\delta ^i}{x^a} = i\left( {\widetilde \varepsilon {\Gamma ^\alpha }\widetilde \theta \not D{{(p_i^{ \pm 1})}^{ - 1/2}}} \right)\gamma _{{a^ \circ }a}^i\sum\limits_{n = - \infty }^\infty {S_{GS}^{10}} \alpha _n^i$

with the Green-Schwarz 10-D action:

$S_{GS}^{10} = \frac{{ - 1}}{{4{\alpha ^ * }\pi }}\int {d\sigma \,d\tau } \left\{ {\sqrt {{g_{\mu \nu }}} {g^{\alpha \beta }}{\prod _\alpha }{\prod ^\beta } + {{\not {\rm Z}}_i}{\varepsilon ^{\alpha \beta }}\not \partial {X^\mu }\left( {{{\widetilde \theta }^1}{\Gamma _{\mu \nu }}\widetilde {\not \partial \,}{\theta ^{ - 1}}{\Gamma _\mu }{{\not \partial }_\beta }{\theta ^2}} \right)} \right\}$

and with $\delta \theta = \varepsilon$ holding. The Vielbein now transforms as:

$\delta {\prod ^a} = - 2\left( {\widetilde \varepsilon {\Gamma ^a}d\theta } \right)$

with:

$\delta d\theta = d\varepsilon$

and:

${\not D_i}{g_{\mu \nu }}(x,\theta ) = d{\widetilde \theta ^\varepsilon }$

where the SuSy covariant derivative is:

${\not D_i}{g_{\mu \nu }} = d{g_{\mu \nu }} - i{g_{\mu \nu }}\left( {d\widetilde \theta {{(p_N^ + )}^{ - 1/2}}\gamma _{{a^ \circ }a}^\nu \sum\limits_{i = - \infty }^\infty {S_{GS}^{10}\alpha _\mu ^i} } \right)$

and solving gives us the p-brane kinetic vibrational action:

$\delta \int {{d^{p + 1}}} \sigma \sqrt {{\rm{detG}}} = - 2i\int {{d^{p + 1}}} \sigma \sqrt {{\rm{detG}}} \left( {\widetilde \varepsilon {\Gamma ^i}{{\not \partial }_i}\theta d\;\Omega {{({\phi _{si}})}^{{e^{ - {\Phi _I}}}}}} \right)$

with ${\Gamma ^i}$ the world-volume gamma matrices derived by a bosonic Yukawa pull-back:

${\Gamma ^i} = \frac{{\not \partial \not {\rm Z}_V^M}}{{\not \partial {\sigma ^i}}}{\prod _a}{\Gamma _a}d\,\Omega {({\phi _{si}})^{{\rm{Bos}}}}$

Now, pulling-back the Lorentz invariant metric ${\eta _{ab}}$ on the bosonic sector of the coset $G/H$ yields the crucial world-volume metric that will solve the ‘initial-Big-Bang-contraction’ problem:

$G_{ij}^{p + 1} = \frac{{\not \partial \not {\rm Z}_V^M}}{{\not \partial {\sigma ^i}}}\frac{{\not \partial \not {\rm Z}_V^N}}{{\not \partial {\sigma ^j}}}{\prod _{{M^a}}}{\prod _{{N^b}}} \equiv {\prod _i}{\prod _j}{\eta _{ab}}$

with ${\sigma ^i}$ the world-volume coordinates. The kinetic p-brane super-term ${S_0}$ , which is proportional to the ‘volume’ of the world-volume, is:

${S_0} = \int {{d^{p + 1}}} \sigma \sqrt {\det G}$

Note that such a bosonic pull-back solves the sparticle vacua degeneracy problem. But, in order to have a solution to ${\not \Delta ^D}\varsigma$ , one must have a Wess-Zumino contribution to the p-brane action with on-shell kappa symmetry:

$\delta \left( {{S_0} + {{\not C}_{WZ}}} \right) = - 4i\int {{d^{p + 1}}} \sigma d\,\Omega {({\phi _{si}})^{p + 1}}\sqrt {\det G} \left( {\widetilde \varepsilon {P_{ \pm \alpha _n^i}}{\Gamma ^i}{{\not \partial }_i}\theta \not D{{\not {\rm Z}}_V}} \right)$

Now, it is key to realize that:

${S_0} + {\not C_{WZ}}$

is kappa symmetric and leads to a coset $G/H$ (p+2)-form:

${h^{p + 2}} = {i^{\left[ {\frac{{p + 1}}{2}} \right]}}\frac{i}{{{P_a}!}}{\prod ^{{a_i}}} \wedge ...{\prod ^{{\alpha _p}}}\left( {d\widetilde {\theta \,}{\Gamma _{{a_1}}}{\Gamma _{{a_2}}}...{\Gamma _{{a_p}}}d\theta \not D{{\not {\rm Z}}_V}} \right)$

The Hilbert Calabi-Yau closure of such a p+2 form is equivalent to the integrability of:

$\delta {\not C_{WZ}} = 2i\int {{d^{p + 1}}} \sigma d{({\phi _{si}})^{p + 1}}\sqrt {\det G} \left( {\widetilde k{\Gamma ^i}{\Gamma _i}\not \partial \theta \not D{{\not {\rm Z}}_V}} \right)$

Now, to solve both problems, we introduce a super-Feynman path term:

$g(t) = \exp \left( {i\left( {{x^a}{P_a} + t + d\widetilde \theta d\,\Omega {{({\phi _{si}})}^{p + t}}} \right)} \right)$

in the fermionic sector of $G/H$ . We then have:

$\frac{d}{{dt}}{\not C_{WZ}}(t) = {i^{\left[ {\frac{{p + 1}}{2}} \right]}}\frac{{2i}}{{P!}}\int {d{\Phi _I}} {\prod _{a(1)}}(t) \wedge ... \wedge {\prod _{a(p)}}\not D{\not {\rm Z}_V}(t){\Gamma _{a(1)}}...{\Gamma _{a(p)}}d\theta \not D{\not {\rm Z}_V}(t)$