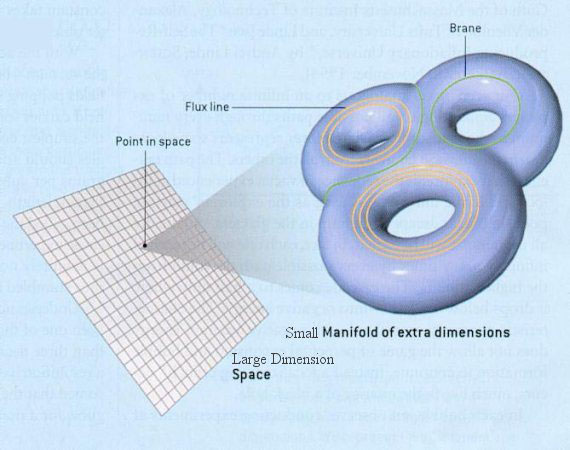
Kappa Symmetry, Dp-Brane Super-Lagrangian Action(s), and SuSy Calabi-Yau ‘Tipping’

By George Shiber Friday, June 26, 2015Sunday, November 3, 2019 In Grand Unification Physics, M Theory, Mathematical Physics, Philosophy Of Mathematics, Philosophy Of Physics, Philosophy Of Science, Super String Theory, Supersymmetry bosons, Branes

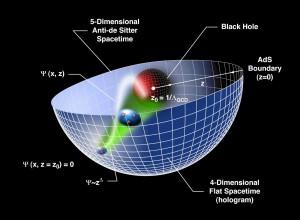
Physicists are more like avant-garde composers, willing to bend traditional rules and brush the edge of acceptability in the search for solutions. Mathematicians are more like classical composers, typically working within a much tighter framework, reluctant to go to the next step until all previous ones have been established with due rigor. Each approach has its advantages as well as drawbacks; each provides a unique outlet for creative discovery. Like modern and classical music, it’s not that one approach is right and the other wrong – the methods one chooses to use are largely a matter of taste and training. ~ Brian Greene!

If supersymmetry is to make touch with reality, not only its ‘breaking‘ needs to be demonstrated, but a mathematically harder hurdle and philosophically deeper matter must be met: and that is to show that a solution to the kappa symmetry algebraic equation:

${\Gamma _\kappa }\left| {_{{\rm{Bo}}{{\rm{s}}^\varepsilon }}} \right. = \varepsilon$

with $B$ the bosonic degrees of freedom, exists and is unique, since that is a necessary condition for a bosonic metaplectic Dp-brane configuration to preserve SuSy, and that would be a sufficient condition for existence, on the bulk, of the SuSy vacua. Whether $B$ has SuSy is equivalent to the existence of a SuSy-transformation with on-shell bosonic symmetry:

$\delta {\not F_{{\rm{Fer}}}}\left| {_{\not F}} \right. = 0$

where $\not F$ is the fermionic degrees of freedom – while leaving:

$\delta {B_{{\rm{bos}}}}\left| {_{{{\not F}_{{\rm{Fer = 0}}}}}} \right. = 0$

intact. Let $\not C$ be the Clifford operator on the bosonic and fermionic fields in the corresponding Hilbert space. Before deriving the dynamics and the action of the SuSy-Hamiltonian, we need to show that:

$\not C({B_{{\rm{bos}}}})\varepsilon = 0$

To simplify, I will work with N=1, D=11 SuGra. Then, the gravitino super-Kähler components:

$\Psi _\alpha ^k = E_\alpha ^M\psi _M^k$

are provably the exact and rigid fermionic degrees of freedom, and transform as:

$\delta \psi _\alpha ^k = \left( {{{\not \partial }_\alpha }{\phi _{si}} + \frac{1}{4}\varpi _\alpha ^{bc}\not \partial {\phi _{si{\Gamma _{bc}}}}} \right)\varepsilon - \frac{1}{{288\pi {i^k}}}\left( {\Gamma _\alpha ^{bcde}} \right)d\,\Omega ({\phi _{si}}){R_{bcde}} \cdot \varepsilon$

with ${\phi _{si}}$ the string variable on the corresponding 2-D world-sheet, and solving:

$\delta \psi _\alpha ^k = 0$

in Dp+2 brane and Dp+5 brane worldvolumes background uniquely induces the Super-Yukawa –Killing spinors on the corresponding super-Lagrangian manifold, with Dp+2 brane being:

$\varepsilon _k^{2\pi i} = {U^{ - 1/6}}{\varepsilon _\infty }{\Gamma _{012}}{\varepsilon _\infty } = \pm {\varepsilon _\infty }$

and for the Dp+5 brane:

$\varepsilon _k^{5\pi i} = {U^{ - 1/12}}{\varepsilon _\infty }{\Gamma _{012345}}{\varepsilon _\infty } = \pm {\varepsilon _\infty }$

The Calabi-Yau sub-space of the bosonic configuration that is determined by $\theta = 0$ condition, where $\theta$ is the Gaillard-Zumino super-Lagrangian torsion, gives us the the total SuSy transformation:

${\delta ^{{\rm{SuSy}}}}\theta \left| {_{{B_{{\rm{bos}}}}}} \right. = 0$

and coupled with:

$d\,{\Omega ^{2\pi ik}}{({\phi _{si}})^2}$

we get:

${\delta ^{{\rm{SuSy}}}}\theta = \delta _k^{{\rm{SuSy}}}\theta \,\not \partial {\phi _{si}}^{2\pi ik} + \varepsilon \,\Delta \theta + {\xi ^\mu }\not \partial \theta d\,\Omega {({\phi _{si}})^2}$

where $\delta _k^{{\rm{SuSy}}}\theta$ is the kappa symmetry diffeomorphism infinitesimal transformation, and:

${\xi ^\mu }{\not \partial _\mu }\theta d\,\Omega {({\phi _{si}})^2}$

is the brane worldvolume diffeomorphism infinitesimal transformation. It is crucial to note that:

$\delta _k^{{\rm{SuSy}}}\theta \left| {_{{B_{{\rm{Bos}}}}}} \right. = \left( {1 + {\Gamma _k}\left| {_{{B_{{\rm{Bos}}}}}d\,\Omega {{({\phi _{si}})}^{2\pi ik}}} \right.} \right) \cdot k$

and:

$\Delta \theta \left| {_{{B_{{\rm{Bos}}}}}} \right. = 0$

both hold. Thus, we get the key identity:

$\delta _k^{{\rm{SuSy}}}\left| {_{{B_{{\rm{Bos}}}}}} \right. = \left( {1 + {\Gamma _k}\left| {_{{B_{{\rm{Bos}}}}}d\,\Omega {{({\phi _{si}})}^{2\pi ik}}{\Delta ^k}\theta \,\not \partial {{({\phi _{si}})}^{2\pi ik}}} \right.} \right) \cdot k$

We are now ready for the Dp-brane Hamiltonian analysis. We start by breaking covariance in order for the time-evolution operator to have a definable Hilbert space spectrum. Let ${\sigma ^\mu } = \left\{ {t,{\sigma ^i}} \right\},{\rm{ }}i = 1,...,p$ be the worldvolume coordinates, and redefine the Dp-brane bosonic super-Lagrangian by extracting out all the time derivatives via momenta conjugation:

${L^{{\rm{SuSy}}}} = {\dot X^m}{P_m} + {\dot V_i}{E^i} + {\dot \psi _{{\rm{Fer}}}}{T_{Dp}} - H\not \partial {({\phi _{si}})^p}$

with $\psi$ the fermionic Hodge dual of the Dp-brane super-Kähler potential, and ${T_{Dp}}$ being the brane tension. So, by quantum fluctuation of the Dp+1 dimensional worldspaces, we get:

$H = {\dot \psi ^{2\pi ik}}{\Im _i} + V_t^{p + 1}\not K + \oint_{p + i} {\delta _k^{{\rm{SuSy}}}} \left| {_{{B_{{\rm{Bos}}}}}} \right.d\,\Omega {({\phi _{si}})^{p + 1}}{\not H_i} + \lambda {\not H^i}$

where:

$\left\{ {\begin{array}{*{20}{c}}{{\Im _i} = - \not \partial {\phi _{si}}{T_{Dp}}d\,\Omega {{({\phi _{si}})}^{2\pi ik}}}\\{\not K = - {{\not \partial }_i}{{\widetilde E}^i} + {{( - 1)}^{p + 1}}{T_{Dp}}{S^{{\rm{Fer}}}}}\\{{{\not H}_i} = \widetilde P{\alpha _i}\widetilde E_i^\alpha {{\not \partial }_i}{\phi _{si}} + \widetilde E{{\not F}_{ij}}}\\{H = \frac{1}{{2\pi ik}}\left[ {{{\widetilde P}^2} + {{\widetilde E}^i}{{\widetilde E}^j}{G_{ij}} + T_{Dp}^2{e^{ - 2{\phi _{si}}}}{\rm{det}}\left( {{G_{ij}} + {{\not F}_{ij}}} \right)} \right]}\end{array}} \right.$

with:

$S = * {\left( {{{\not R}_{\mu \nu }}{\varepsilon ^{{\rm{Fer}}}}} \right)_p}$

and:

$E_i^\alpha = \delta \int {d\not E_m^\alpha } {\not \partial _i}{\dot X^m}$

Solving gives us the Dp+2 brane Lagrangian:

$L_D^{p + 2} = {\dot X^m}{P_m}\not \partial {({\phi _{si}})^{2\pi ik}}\delta _k^{{\rm{SuSy}}}{\widetilde P_\alpha }E_i^\alpha d\,\Omega {({\phi _{si}})^{2\pi ik}} \cdot \frac{1}{{2\pi ik}}\lambda \left[ {{{\widetilde P}^2} + T_{Dp + 2}^2{\rm{det}}{{\rm{G}}_{ij}}} \right]$

with:

${\widetilde P_\alpha } = E_\alpha ^m\left( {{P_m} + {T_{Dp + 2}}C_m^{Dp + 2}} \right)$

and:

$C_m^{Dp + 2} = * {\left( {{Z^ * }({i_m}\not C({B_{{\rm{Bos}}}})} \right)^2}$

and similarly for the Dp+5 brane with appropriate numerical ‘substitutions’. Solving both, in the fermionic and bosonic sectors of the super-Lagrangian manifold, one gets, by integrating over Dp+n worldspaces, n=2, 5, the SuSy vacua that generate the complete set of superpartners, and is:

${\not Z_V} = {T_{M_L^{Dp + n}}}dy \wedge dz = {T_{{M^{Dp + n}}}}\frac{i}{2}\int_{M_L^{Dp + n}} {d\,\Omega } {({\phi _{si}})^{p + n}} \wedge d\,\widetilde \Omega {({\phi _{si}})^{ - p + n}}$

Solving it induces a kappa symmetric super-Lagrangian SuSy vacua that embeds the superpartner fields on the conic tip of a Calabi-Yau manifold whose tangent bundle is isomorphic to:

$Ad{S_5} \times E_S^5$