Clifford-Kähler Algebras and M-Theory Compactification

By George Shiber Friday, January 20, 2017Saturday, August 17, 2019 In Cosmology, Grand Unification Physics, M Theory, Mathematical Physics, Mathematics, Physics, Super String Theory, Supersymmetry Clifford-Kähler Algebras, Kähler-Atiyah Algebras

Continuing from my work on the relation between Clifford algebraic symmetries and M-theory, here I will initiate an analysis of compactification via the derived Kähler-Atiyah bundle associated with Clifford-Kähler manifolds. Recall that whenever 2 or more D-branes coincide, there is a Clifford algebraic symmetry whose generators allow us to derive the total action:

$\begin{array}{*{20}{l}}{{S^{Total}} = \frac{1}{{2\pi {\alpha ^\dagger }12}}\int\limits_{{\rm{world - volumes}}} {{d^{26}}} x{\mkern 1mu} d{\mkern 1mu} \Omega {{\left( {{\phi _{Inst}}} \right)}^2}\sqrt {\frac{{ - {g_{\mu \nu }}}}{{ - \gamma }}} {\mkern 1mu} {e^{ - {c_{2n}}/{\Upsilon _\kappa }(\cos \varphi )}} \cdot }\\{\left( {{R_{icci}} - 4{{\left( {{{\not D}^{SuSy}}\left( {{\phi _{Inst}}} \right)} \right)}^2}} \right) + \frac{1}{{12}}H_{3,\mu \nu \lambda }^bH_3^{b,\mu \nu \lambda }/A_\mu ^H + \sum\limits_{D - p - branes} {S_{Dp}^{WV}} }\end{array}$

and since D-p-branes are metaplectic solitons in closed string-theory, by the von Neumann boundary condition, there is a natural coupling of the super-Higgs field $A_\mu ^H$ to the world-sheet of a string through its boundary:

${S_{open}} = {S_{cld}} + \int\limits_{{\rm{end - points}}} {d\tau } A_\mu ^H{\bar X^\mu }{e^{ - H_3^b}}d{\mkern 1mu} \Omega {\left( {{\phi _{Inst}}} \right)^{\exp {\kern 1pt} ({c_{2n}}/{\Upsilon _\kappa }(\cos \varphi )}}$

Hence, $A_\mu ^H$ lives on a p+1 dimensional subspace with a ${\Upsilon _\kappa }(\cos \varphi )$ contribution, yielding the world-volume action:

$S_{Dp}^{WV} = {S_{cld}} + \int\limits_{{\rm{end - points}}} {d\tau } A_\mu ^H{\bar X^\mu }d{\mkern 1mu} \Omega {\left( {{\phi _{Inst}}} \right)^2}{e^{ - \left( {H_3^b} \right)/{\Upsilon _\kappa }(\cos \varphi )}} + {e^{{c_{2n}}/{\Upsilon _\kappa }(\cos \varphi )}}/H_3^b$

and since world-volumes have conformal invariance, by solving the n-loop level equation of motion:

$\left\{ {\begin{array}{*{20}{c}}{{R_{\mu \nu }} = - \frac{1}{4}H_{3,\mu \lambda l}^bH_{3,\nu }^{b,\lambda \rho } + 2{{\not D}_\mu }^S{{\left( {{\phi _{Inst}}} \right)}^2}{{\not D}_\nu }^S{{\left( {{\phi _{Inst}}} \right)}^{1/2}}}\\{{{\not D}_\lambda }^S{\phi _{Inst}}H_{3,\lambda \mu \nu }^b - 4{{\not D}_\mu }^S{{\left( {{\phi _{Inst}}} \right)}^2}}\\{4{{\not D}_l}^S{\phi _{Inst}} - 4\left( {{{\not D}_\mu }^S{{\left( {{\phi _{Inst}}} \right)}^2}} \right) = {R_{icci}} + \frac{1}{{12}}{{\left( {H_3^b} \right)}^2}}\end{array}} \right.$

we get the total world-volume action:

$\begin{array}{*{20}{c}}{S_{Dp}^{WV} = {\mkern 1mu} - {T_p}\int {{d^{p + 1}}} x{\mkern 1mu} {e^{ - {\phi _{Inst}}}}{\rm{Tr}}\left( {1 + \frac{1}{4}\left( {{F_{\mu \nu }} + d{\mkern 1mu} \Omega {{\left( {{\phi _{Inst}}} \right)}^2}} \right) + {{\left( {2\pi {\alpha ^\dagger }} \right)}^{ - 1}}{b_{\mu \nu }}} \right) \cdot }\\{\left( {A_\mu ^H + {{\left( {2\pi {\alpha ^\dagger }} \right)}^{ - 1}}{b_{\mu \nu }}} \right) + \frac{1}{2}{{\not D}^S}{\Upsilon _\kappa }(\cos \varphi ) - \frac{1}{4}\sum\limits_{i \times j} {\left[ {\Upsilon _{2\kappa }^i(\cos \varphi ,\Upsilon _{2\kappa }^j(\cos \varphi } \right]} \cdot }\\{\left[ {\Upsilon _{2\kappa }^j(\cos \varphi ),\Upsilon _{2\kappa }^i(\cos \varphi )} \right]}\end{array}$

and since Clifford algebras are a quantization of target-space exterior algebras, via Gaussian matrix-elimination, we can expand, via Green’s-function, ${\not D^{SuSy}}$ , the supersymmetry group covariant derivative, which I have redundantly simplified to ${\not D^S}$ and by supersymmetry, the total action of M-Theory becomes:

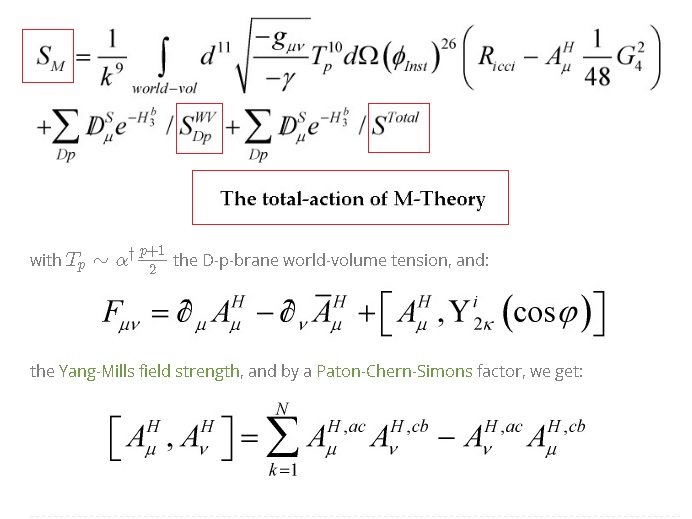
$\begin{array}{*{20}{l}}{{S_M} = \frac{1}{{{k^9}}}\int\limits_{world - vol} {{d^{11}}} \sqrt {\frac{{ - {g_{\mu \nu }}}}{{ - \gamma }}} T_p^{10}d\Omega {{\left( {{\phi _{Inst}}} \right)}^{26}}\left( {{R_{icci}} - A_\mu ^H\frac{1}{{48}}G_4^2} \right)}\\{ + \sum\limits_{Dp} {\not D_\mu ^S} {e^{ - H_3^b}}/S_{Dp}^{WV} + \sum\limits_{Dp} {\not D_\mu ^S} {e^{ - H_3^b}}/{S^{Total}}}\end{array}$

with ${T_p} \sim {\alpha ^\dagger }\frac{{p + 1}}{2}$ the D-p-brane world-volume tension, and:

${F_{\mu \nu }} = {\partial _\mu }A_\mu ^H - {\partial _\nu }\bar A_\mu ^H + \left[ {A_\mu ^H,\Upsilon _{2\kappa }^i(\cos \varphi )} \right]$

the Yang-Mills field strength, and by a Paton-Chern-Simons factor, we get:

$\left[ {A_\mu ^H,A_\nu ^H} \right] = \sum\limits_{k = 1}^N {A_\mu ^{H,ac}} A_\nu ^{H,cb} - A_\nu ^{H,ac}A_\mu ^{H,cb}$

${\phi _{Inst}}$ the instanton field, with:

${e^{ - {\phi _{Inst}}{g_{\mu \nu }}}} = {e^{ - 2{\phi _{Inst}}\left( {{g_{\mu \nu }} - 1} \right)}}$

here: ${g_{\mu \nu }} = {e^{{{\left( {{\phi _{Inst}}} \right)}^2}}}$ .

In N=2 compactifications of 11-dimensional supergravity down to a 3-dimensional (anti)-deSitter space, the internal eight-D-manifold $M$ comes equipped with a Riemannian metric $g$ and a Kähler 1-form $f$ and a 4-form $F$ encoding the 4-form field strength of the 11-dimensional theory. Moreover, the Majorana spinor is a section of the real spin bundle $S$ of $M$ . The background conserves exactly N=2 supersymmetry in 3-dimensions, equivalent to the condition that the real vector-space of solutions to the following differentio-algebraic system of generalized Killing spinor equations:

${D^K}\xi ' = Q\xi ' = 0$

is two-dimensional, with $Q$ an endomorphism of $S$ :

$\begin{array}{l}Q = \frac{1}{2}{{\tilde \gamma }^m}{\partial _m}\Delta - \frac{1}{{288}}{F_{mpqr}}{{\tilde \gamma }^{mpqr}}\\ - \frac{1}{6}{f_{\hat P}}{{\tilde \gamma }^{\hat P}}{{\tilde \gamma }^{\left( 9 \right)}} - \kappa {{\tilde \gamma }^{\left( 9 \right)}}\end{array}$

${D^K} = {\nabla ^S}$ the Kähler connection on $S$ , and ${\nabla ^S}$ is the connection induced on $S$ by the Levi-Civita connection of $\left( {M,g} \right)$ where:

$A = {\rm{d}}{x^m} \otimes {A_m} \in {\Omega ^1}\left( {M,{\rm{End}}\left( S \right)} \right)$

is an ${\rm{End}}\left( S \right)$ -valued 1-form on $M$ , with:

$\begin{array}{c}{A_m} = \frac{1}{4}{f_{\hat P}}{{\tilde \gamma }_m}^{\hat P}{{\tilde \gamma }^{\left( 9 \right)}} + \frac{1}{{24}}{F_{mpqr}}{{\tilde \gamma }^{pqr}}\\ + \kappa {{\tilde \gamma }_m}{{\tilde \gamma }^{\left( 9 \right)}} \in \Gamma \left( {{\rm{End}}\left( S \right)} \right)\end{array}$

with $\kappa$ related to the cosmological constant $\Lambda$ of ${\rm{Ad}}{{\rm{S}}_3}$ as:

$\Lambda = - 8{\kappa ^2}$

The F-motivated centrality of the chirality constraint:

${\tilde \gamma ^{\left( 9 \right)}}\xi ' = + \xi$

allows the construction of the spin bundle $S$ of a (pseudo)-Riemannian manifold $\left( {M,g} \right)$ with signature $\left( {p,q} \right)$ of dimension $d = p + q$ describable by an $S$ -bundle of modules over the Clifford bundle ${\rm{CL}}\left( {{T^ * }M} \right)$ of the cotangent bundle of ${{T^ * }M}$ endowed with a metric $\hat g$ induced by ${g^ * }$ . The initial problem is that the Clifford bundle is determined by $\left( {M,g} \right)$ only up to isomorphism, so the association of ${\rm{CL}}\left( {{T^ * }M} \right)$ to $\left( {M,g} \right)$ is not functorial. One typically goes around this problem by the invoking the Kähler-Atiyah Clifford-realization bundle of $\left( {M,g} \right)$ .

This is the Chevalley-Riesz realization and it yields:

${\rm{Cl}}\left( {{T^ * }M} \right) \cong \wedge {T^ * }M$

where $\wedge {T^ * }M$ is the exterior algebra of $M$ and the Clifford product of ${\rm{CL}}\left( {{T^ * }M} \right)$ is non-commutative, and the fiberwise multiplication on $\wedge {T^ * }M$ : $^\circ$ , transforms $\wedge {T^ * }M$ into the Kähler-Atiyah bundle $\left( { \wedge {T^ * }M, \circ } \right)$ .

Hence, the corresponding $\mathbb{Z}$ -grading admits an expansion into a finite sum of homogeneous-degree $- 2\kappa$ binary operations ${\Delta _k},\;k = 0,...,d$ satisfying:

and the parity automorphism $\pi$ is given by:

$\pi \overbrace = ^{def.} \otimes _{k = 0}^d{\left( { - 1} \right)^k}{\rm{i}}{{\rm{d}}_{{ \wedge ^k}{T^ * }M}}$

with:

${\Delta _k}: \wedge {T^ * }M{ \times _M} \wedge {T^ * }M \to {T^ * }M$

the Kähler-Atiyah generalized products.

Hence, the expansion:

is a semiclassical expansion of the geometric product yielding a geometric quantization where the Planck constant is inversely related to the scale of the metric $g$ , thus allowing us to derive:

$\begin{array}{c}\omega {\Delta _{k + 1}}\eta = \frac{1}{{k + 1}}{g^{ab}}\left( {{e_a}{,_\parallel }\omega } \right){\Delta _k}\left( {{e_a}{,_\parallel }\eta } \right)\\ = {g_{ab}}\left( {{\iota _{{e^b}}}\eta } \right)\end{array}$

with $\iota$ the interior product.

Hence, our spin bundle $S$ can be interpreted as a bundle of modules over the Kähler-Atiyah bundle of $\left( {M,g} \right)$ , with the module structure defined by a morphism of bundle of algebras:

$\tilde \gamma :\left( { \wedge {T^ * }M, \circ } \right) \to \left( {{\rm{End}}\left( S \right), \circ } \right)$

where $\tilde \gamma$ is fiberwise-irreducible.

With ${\left( {{e_m}} \right)_{m = 1...8}}$ the local $TM$ -frame, ${\left( {{e^m}} \right)^{m...8}}$ the dual ${T^ * }M$ -co-frame, satisfying:

$\left\{ {\begin{array}{*{20}{c}}{{e^m}\left( {{e_n}} \right) = \delta _n^m}\\{\hat g\left( {{e^m},{e^n}} \right) = {g^{mn}}}\end{array}} \right.$

with:

$\Omega \left( M \right)\overbrace = ^{{\rm{def}}}\Gamma \left( {M, \wedge {T^ * }M} \right)$

the space of smooth inhomogeneous globally defined differential forms on $M$ , and a form $\omega$ expands as:

$\omega = \sum\limits_{k = 0}^d {{\omega ^{\left( k \right)}}} { = _U}\sum\limits_{k = 0}^d {\frac{1}{{k!}}\omega _{{a_1}...{a_k}}^{\left( k \right)}} {e^{{a_1}...{a_k}}}$

${\omega ^{\left( k \right)}} \in {\Omega ^k}\left( M \right)$

with:

${e^{{a_1}...{a_k}}}\overbrace = ^{{\rm{def}}}{e^{{a_1}}} \wedge ... \wedge {e^{{a^k}}}$

A real spinors-bundle over $M$ is an $\mathbb{R}$ -vector bundle $S$ over $M$ that is also a bundle of modules over the Clifford bundle ${\rm{Cl}}\left( {{T^ * }M} \right)$ .

Since we have no loss of generality by assuming that $M$ is orientable with $\nu$ the volume form satisfying $\nu \circ \nu = + 1$ , the bundle decomposition is hence:

$\wedge {T^ * }M = {\left( { \wedge {T^ * }M} \right)^ + } \oplus {\left( { \wedge {T^ * }M} \right)^ - }$

and the ${C^\infty }\left( {M,\mathbb{R}} \right)$ -submodules of $\Omega \left( M \right)$ yield:

$\begin{array}{l}{\Omega ^ \pm }\left( M \right)\overbrace = ^{{\rm{def}}}\Gamma \left( {M,{{\left( { \wedge {T^ * }M} \right)}^ \pm }} \right) = \\\left\{ {\omega \in \Omega \left( M \right)\left| {\omega \circ \nu = \pm \omega } \right.} \right\}\end{array}$

as well as:

${\left( { \wedge {T^ * }M} \right)^\gamma }\overbrace = ^{{\rm{def}}}\left\{ {\begin{array}{*{20}{c}}{ \wedge {T^ * }M,{\kern 1pt} \;d{ \ne _8}1,5}\\{{{\left( { \wedge {T^ * }M} \right)}^{ + \varepsilon \gamma }},\;d{ \equiv _8}1,5}\end{array}} \right.$

where $\gamma$ is implicitly defined by:

$\gamma \left| {_{{{\left( { \wedge {T^ * }M} \right)}^{ - \varepsilon \gamma }}}} \right. = 0$

which is a surjective mapping, and since Clifford algebras are a quantization of target-space exterior algebras of the Kähler-Atiyah bundle, the Kähler-Atiyah algebra is hence:

${\Omega ^\gamma }\left( M \right)\overbrace = ^{{\rm{def}}}\Gamma \left( {M,{{\left( {{T^ * }M} \right)}^\gamma }} \right)$

Without any loss of generality, we can stick to dealing with $M$ a Riemannian 8-manifold that is the compactification space of M-theory down to 3 dimensions or a 9-manifold corresponding to the metric cone over an eight-dimensional compactification space. Hence, the fiberwise representation given by $\gamma$ is equivalent to an irreducible representation of the real Clifford algebra ${\rm{CL}}\left( {8,0} \right)$ or ${\rm{CL}}\left( {9,0} \right)$ in a 16-dimensional $\mathbb{R}$ -vector space, which is surjective, and thus we have:

${\tilde \gamma ^{ - 1}}\overbrace = ^{{\rm{def}}}{\left( {\tilde \gamma \left| {_{{{\left( { \wedge {T^ * }M} \right)}^{\tilde \gamma }}}} \right.} \right)^{ - 1}}:{\rm{End}}\left( S \right) \to {\left( { \wedge {T^ * }M} \right)^{\tilde \gamma }}$

which is a map that identifies the bundle of endomorphisms of $S$ with the bundle of algebras:

$\left( {{{\left( { \wedge {T^ * }M} \right)}^{\tilde \gamma }}, \circ } \right)$

and so every globally-defined endomorphism:

$T \in \Gamma \left( {M,{\rm{End}}\left( S \right)} \right)$

admits a dequantization:

$\mathord{\buildrel{\lower3pt\hbox{$\scriptscriptstyle\smile$}} \over T} \overbrace = ^{{\rm{def}}}{\tilde \gamma ^{ - 1}}\left( T \right) \in {\Omega ^{\tilde \gamma }}\left( M \right)$

that defines a differential form on $M$ , yielding ${T_1} \circ {T_2} = {\mathord{\buildrel{\lower3pt\hbox{$\scriptscriptstyle\smile$}} \over T} _1} \circ {\mathord{\buildrel{\lower3pt\hbox{$\scriptscriptstyle\smile$}} \over T} _2}$ of the dequantizations of ${T_1},{T_2} \in \Gamma \left( {M,{\rm{End}}\left( S \right)} \right)$ .

We can now define the Fierz isomorphism of bundles of algebras:

$\mathord{\buildrel{\lower3pt\hbox{$\scriptscriptstyle\smile$}} \over E} :\left( {S \otimes S, \bullet } \right)\widetilde \to \left( {{{\left( { \wedge {T^ * }M} \right)}^{\tilde \gamma }}, \circ } \right)$

with:

$\left( {S \otimes S, \bullet } \right)$

the bi-spinor bundle. On fiber sections, we hence have an isomorphism of ${C^\infty }\left( {M,\mathbb{R}} \right)$ -algebras:

$\mathord{\buildrel{\lower3pt\hbox{$\scriptscriptstyle\smile$}} \over E} :\left( {\Gamma \left( {M,S \otimes S} \right) \bullet } \right)\widetilde \to \left( {{\Omega ^{\tilde \gamma }}\left( M \right), \circ } \right)$

identifying the bi-spinor algebra $\left( {\Gamma \left( {M,S \otimes S} \right) \bullet } \right)$ with the subalgebra $\left( {{\Omega ^{\tilde \gamma }}\left( M \right), \circ } \right)$ of the Kähler-Atiyah algebra.

Let us now define the inhomogeneous differential forms:

$\left\{ {\begin{array}{*{20}{c}}{{{\mathord{\buildrel{\lower3pt\hbox{$\scriptscriptstyle\smile$}} \over E} }_{\xi ,\xi '}}\overbrace = ^{{\rm{def}}}\mathord{\buildrel{\lower3pt\hbox{$\scriptscriptstyle\smile$}} \over E} \left( {\xi \otimes \xi '} \right) \in {\Omega ^{\tilde \gamma }}\left( M \right)}\\{\xi ,\xi ' \in \Gamma \left( {M,S} \right)}\end{array}} \right.$

Here is where Clifford-Kähler manifolds come in. The corresponding properties of the Fierz isomorphism, the algebraic constraint $Q\xi = 0$ and the generalized Killing spinor equations ${D^K}\xi = 0$ yield the following conditions on the inhomogeneous differential forms ${\mathord{\buildrel{\lower3pt\hbox{$\scriptscriptstyle\smile$}} \over E} _{\xi ,\xi '}}$ , which hold for any global section:

$\xi ,\xi ' \in \Gamma \left( {M,S} \right)$

satisfying:

${D^K}\xi ' = Q\xi ' = 0$

${\mathord{\buildrel{\lower3pt\hbox{$\scriptscriptstyle\smile$}} \over D} ^{{K^{{\rm{ad}}}}}}{\mathord{\buildrel{\lower3pt\hbox{$\scriptscriptstyle\smile$}} \over E} _{\xi ,\xi '}} = \mathord{\buildrel{\lower3pt\hbox{$\scriptscriptstyle\smile$}} \over Q} \circ {\mathord{\buildrel{\lower3pt\hbox{$\scriptscriptstyle\smile$}} \over E} _{\xi ,\xi '}} = 0$

where the dequantization of the globally defined endomorphism

${Q \in \Gamma \left( {M,{\rm{End}}\left( S \right)} \right)}$

and

${{{\mathord{\buildrel{\lower3pt\hbox{$\scriptscriptstyle\smile$}} \over D} }^{{K^{{\rm{ad}}}}}} = {e^m} \otimes {{\mathord{\buildrel{\lower3pt\hbox{$\scriptscriptstyle\smile$}} \over D} }^{{K^{{\rm{ad}}}}}}}$

the adjoint dequantization, is:

$\mathord{\buildrel{\lower3pt\hbox{$\scriptscriptstyle\smile$}} \over Q} \overbrace = ^{{\rm{def}}}{\tilde \gamma ^{ - 1}}\left( Q \right) \in \Omega \left( M \right)$

and:

$\mathord{\buildrel{\lower3pt\hbox{$\scriptscriptstyle\smile$}} \over D} _m^{{K^{{\rm{ad}}}}}$

are the Kähler-Atiyah derivatives given by:

$\mathord{\buildrel{\lower3pt\hbox{$\scriptscriptstyle\smile$}} \over D} _m^{{K^{{\rm{ad}}}}}\overbrace = ^{{\rm{def}}}{\nabla _m} + {\left[ {{{\mathord{\buildrel{\lower3pt\hbox{$\scriptscriptstyle\smile$}} \over A} }_{m,...}}} \right]_{ - , \circ }}$

with $\nabla$ the Levi-Civita connection of $\left( {M,g} \right)$ induced on $\wedge {T^ * }M$ , which yield us the Fierz identities:

${\mathord{\buildrel{\lower3pt\hbox{$\scriptscriptstyle\smile$}} \over E} _{{\xi _1},{\xi _2}}} \circ {\mathord{\buildrel{\lower3pt\hbox{$\scriptscriptstyle\smile$}} \over E} _{{\xi _3},{\xi _4}}} = \Im \left( {{\xi _3},{\xi _2}} \right){\mathord{\buildrel{\lower3pt\hbox{$\scriptscriptstyle\smile$}} \over E} _{{\xi _1},{\xi _4}}}$

that define, via Clifford-Kähler fibration, the Kähler-Atiyah sub-algebra of $\left( {M,g} \right)$ .

Now, this class of equation:

lifts $\xi$ to the metric cone over $M$ , which can be interpreted as the warped product:

$\left( {\hat M,{g_{cone}}} \right) \approx \left( {\left( {0,\infty } \right),{\rm{d}}{\tau ^2}} \right){ \times _\tau }$

with warp factor:

${\rm{d}}s_{cone}^2 = {\rm{d}}{\tau ^2} + {\tau ^2}{\rm{d}}{s^2}$

with the corresponding Kähler 1-form:

$\theta \overbrace = ^{{\rm{def}}}{\rm{d}}\tau = {\partial _{{\tau ^\parallel }}}{g_{cone}}$

We can now identify the spin bundle $\hat S$ of the metric cone over $M$ with the pullback of $S$ through the natural projection:

$\Pi :\hat M \to M$

by defining the lift ${\hat D^K}$ of ${D^K}$ to be the connection on $\hat S$ obtained via ${D^K}$ on the pullback to the cone. Hence, ${\hat D^K}$ can be written as:

${\hat D^K} = {\nabla ^{\hat S,cone}} + {A^{cone}}$

where ${\nabla ^{\hat S,cone}}$ is the $\hat S$ -connection induced by the Levi-Civita connection of ${g_{cone}}$ . Hence, the Schur algebra associated with our Clifford algebra ${\rm{Cl}}\left( {9,0} \right)$ is isomorphic to $\left( {\hat M\left( {\hat S} \right),{g_{cone}}} \right)$ and

the corresponding spin representation:

${\tilde \gamma _{cone}}:\left( { \wedge {T^ * }\hat M,{ \circ _{cone}}} \right) \to \left( {{\rm{End}}\left( {\hat S} \right), \circ } \right)$

is surjective. The morphism ${\tilde \gamma _{cone}}$ is completely determined once the signature $\varepsilon$ is fixed. With no loss of generality, we can take it to be: $\varepsilon = + 1$ , and by a shift-rescaling, on $M$ , of the metric:

$g \to {\left( {2\kappa } \right)^2}g$

we can deduce the conical equations:

$\nabla _m^{\hat S,cone} = \nabla _m^S + \kappa {\tilde \gamma _{m9}}$

$A_9^{cone} = 0$

and

$A_m^{cone} = \frac{1}{4}{f^p}{\tilde \gamma _{mp9}} + \frac{1}{{24}}{F_{mpqr}}{\tilde \gamma ^{pqr}}$

And crucially, the Killing spinor equations:

${D^K}_m\xi = 0$

for spinors defined on $M$ :

$\xi \in \Gamma \left( {M,S} \right)$

for m = 1 … 8, yield the Clifford-Kähler manifold flatness conditions:

$\left\{ {\begin{array}{*{20}{c}}{{{\hat D}_a}\hat \xi = 0}\\{\forall a = 1...9}\end{array}} \right.$

$\forall \hat \xi \in \Gamma \left( {\hat M,\hat S} \right)\left| {_{{\rm{def}}\left( {\hat M} \right)}} \right.$

Notice also, that the conic-flatness equation:

${\hat D^K}\hat \xi = 0$

is equivalent to the condition that the section $\hat \xi$ of $\hat S$ is the Clifford-pullback of a section $\xi$ of $S$ through the Kähler-Atiyah projection $\Pi$ from $\hat M$ to $M$ , and the remaining equations are fundamentally the generalized Killing conditions:

${D^K}_m\hat \xi = 0\left| {_{ \in M}} \right.$

Thus, the Kähler-Atiyah algebra of cones generalizes Killing spinors on the Clifford-Kähler algebras and after fribrations, we obtain the desired N=2 compactifications of M-theory down to three dimensions conified on:

$\Upsilon _{2\kappa }^{Q\left[ {\hat M\left( {\hat S} \right)} \right]}$