Continuing from where I left off revealing paradoxical aspects of wave-function collapse involving time-reversal symmetry and the quantum entanglement of the total system consisting of ‘S’, ‘m’ and the quantum-time measuring clock ‘c’ subject to Heisenberg’s UP, consider the collapse equation:
![Rendered by QuickLaTeX.com \[\begin{array}{*{20}{l}}{d{\psi _t}^{S,m,c} = \left[ { - iHdt + \sum\limits_{k = 1}^n {\left( {{L_k} - {\ell _{k,t}}} \right)} } \right.}\\{d{W_{k,t}} - \frac{1}{2}\sum\limits_{k = 1}^n {\left. {\left( {L_k^\dagger {L_k} - 2{\ell _{k,t}}{L_k} + {{\left| {{\ell _{k,t}}} \right|}^2}} \right)} \right]} }\\{{\psi _t}^{S,m,c}}\end{array}\]](https://www.georgeshiber.com/wp-content/ql-cache/quicklatex.com-1a83933a29c2181918c761122e6c8710_l3.png)
with ![]() the Hilbert-space Lindblad collapse operators,
the Hilbert-space Lindblad collapse operators, ![]() given by:
given by:
![]()
and the first term on the right-hand side represents the unitary quantum evolution, the rest the collapse of the wave-function. Dynamically, to ensure the positivity of the reduced density matrix:
![]()
the Lindblad collapse equation:
![Rendered by QuickLaTeX.com \[\begin{array}{*{20}{l}}{d\left| {{\psi _t}^{S,m,c}} \right\rangle = \left[ { - \frac{i}{\hbar }} \right.Hdt + \sqrt \lambda \int {{d^3}} x\left( {N(\bar x) - {{\left\langle {N(\bar x)} \right\rangle }_t}} \right)}\\{d{W_t}(\bar x) - \frac{\lambda }{2}\int {{d^3}} x\left. {{{\left( {N(\bar x) - {{\left\langle {N(\bar x)} \right\rangle }_t}} \right)}^2}dt} \right]\left| {{\psi _t}^{S,m,c}} \right\rangle }\end{array}\]](https://www.georgeshiber.com/wp-content/ql-cache/quicklatex.com-f089a61dd392ed76a116681960c0ba6e_l3.png)
would entail that the Lindblad quantum-jump equation:
![Rendered by QuickLaTeX.com \[\begin{array}{l}{\rm{d}}\hat \rho _S^C = - i\left[ {{{\hat H}_S},\hat \rho _S^C} \right]{\rm{d}}t - \\\frac{1}{2}\sum\limits_\mu {{\kappa _\mu }} \left[ {{{\hat L}_\mu },\left[ {{{\hat L}_\mu },\hat \rho _S^C} \right]} \right]{\rm{d}}t + \\\sum\limits_\mu {\sqrt {{\kappa _\mu }} } W\left[ {{{\hat L}_\mu }} \right]\hat \rho _S^C{\rm{d}}{W_\mu }\end{array}\]](https://www.georgeshiber.com/wp-content/ql-cache/quicklatex.com-ee87490921747ac619bc820689a9b67a_l3.png)
is solvable iff ![]() commutes with the position-operator, with:
commutes with the position-operator, with:
![Rendered by QuickLaTeX.com \[\begin{array}{l}W\left[ {\hat L} \right]\hat \rho \equiv \hat L\hat \rho + \hat \rho {{\hat L}^\dagger } - \\\hat \rho {\rm{Tr}}\left\{ {\hat L\hat \rho + \hat \rho {{\hat L}^\dagger }} \right\}\end{array}\]](https://www.georgeshiber.com/wp-content/ql-cache/quicklatex.com-ce95b689b7da81151afd2ddb7cc775b3_l3.png)
where ![]() is the the Markovian reduced density operator, and
is the the Markovian reduced density operator, and ![]() the Weiner-quantum-increments.
the Weiner-quantum-increments.
However:
![]()
commutes with the energy operator. By the Heisenberg energy-time uncertainty principle, the collapse equation cannot be integrated to get the collapse-localization double-integral in 4-D:
![]()
Now applying the wave-particle duality to:
![]()
implies therefore, by the time-local non-Markovian master equation:
![]()
that the Lindblad quantum-jump equation is ill-defined over any quantum state describing the total system: in particular, a quantum-time measuring clock (QTMC). This much is guaranteed by the Heisenberg uncertainty principle relating time and energy. Thus, since such a QTMC is essential for Schrödinger’s equation, the paradox is now clear.
Let us look at how quantum Markovian jumps can offer a decoherence way out. Let a system S be described by the master equation for the reduced density operator in the Markovian approximation:
![Rendered by QuickLaTeX.com \[\begin{array}{c}\dot \rho = i\left[ {\hat H,\rho } \right] + \sum\limits_m {{{\hat L}_m}} \hat L_m^\dagger - \frac{1}{2}\hat L_m^\dagger {{\hat L}_m}\rho \\ - \frac{1}{2}\rho \hat L_m^\dagger {{\hat L}_m}\end{array}\]](https://www.georgeshiber.com/wp-content/ql-cache/quicklatex.com-29f800ed3ba0e5b71e45fbb966a689e6_l3.png)
which has a Heisenberg-cut unraveling into a quantum jump process for pure states, with the usual Hamiltonian and the Lindblad operators which describe the effects of the environment are the set ![]() . Here, I am describing quantum systems via an exhaustive set of possible histories that satisfy a decoherence or consistency-histories criterion obeying the classical probability sum rules. Moreover, such quantum jumps are interpreted as yielding the values of continuous measurements and the histories correspond to records of a classical measuring device that must decohere. Hence, there is a set of decoherent histories that correspond to the quantum trajectories of a continuously measured system. See Ian Percival as well as Lajos Diósi for such remarkable correspondences.
. Here, I am describing quantum systems via an exhaustive set of possible histories that satisfy a decoherence or consistency-histories criterion obeying the classical probability sum rules. Moreover, such quantum jumps are interpreted as yielding the values of continuous measurements and the histories correspond to records of a classical measuring device that must decohere. Hence, there is a set of decoherent histories that correspond to the quantum trajectories of a continuously measured system. See Ian Percival as well as Lajos Diósi for such remarkable correspondences.
Now take a quantum system with a Hamiltonian ![]() with complete single channel of decay tracked by an external photon detector described by a model of a single 2-level system with states
with complete single channel of decay tracked by an external photon detector described by a model of a single 2-level system with states ![]() and
and ![]() strongly coupled to an environment representing the remaining degrees of freedom. The two crucial properties are clearly, 1) dissipation: Excitations of the states absorbed by the measuring device with a rate
strongly coupled to an environment representing the remaining degrees of freedom. The two crucial properties are clearly, 1) dissipation: Excitations of the states absorbed by the measuring device with a rate ![]() , with the time-resolution of the detector given by
, with the time-resolution of the detector given by ![]() , and 2) decoherence: As output mode states become correlated with the internal degrees of freedom of the measuring device, the phase coherence between the ground and excited states of the output mode is completely and tracelessly lost,
, and 2) decoherence: As output mode states become correlated with the internal degrees of freedom of the measuring device, the phase coherence between the ground and excited states of the output mode is completely and tracelessly lost,
and the loss of coherence is far quicker than the actual rate of energy loss, with decoherence rate 
So, the linearized system coupled to the output mode via the Hamiltonian:
![]()
with the following total Hamiltonian:
![]()
with ![]() the lowering/raising operators for the system and output mode respectively entails that the total system satisfies the master equation:
the lowering/raising operators for the system and output mode respectively entails that the total system satisfies the master equation:
![Rendered by QuickLaTeX.com \[\begin{array}{c}\dot \rho = - i\left[ {\hat H,\rho } \right] + {\Gamma _1}\hat b\rho {{\hat b}^\dagger } - \frac{{{\Gamma _1}}}{2}{{\hat b}^\dagger }\hat b\rho \\ - \frac{{{\Gamma _1}}}{2}\rho {{\hat b}^\dagger }\hat b + {\Gamma _2}{\sigma _z}\rho {\sigma _z} - {\Gamma _2}\rho \\ \equiv L_s^L\rho \end{array}\]](https://www.georgeshiber.com/wp-content/ql-cache/quicklatex.com-cc3758ebaace5c12297a8b89dc8bcc3f_l3.png)
where the Pauli operator ![]() acts on the output mode and
acts on the output mode and ![]() is the Liouville superoperator. Given that it is a linear equation, it has a solution given as:
is the Liouville superoperator. Given that it is a linear equation, it has a solution given as:
![]()
Start with a pure state:
![]()
and expand ![]() as:
as:
![Rendered by QuickLaTeX.com \[\begin{array}{l}\rho (t) = {\rho _{00}}(t) \otimes \left| 0 \right\rangle \left\langle 0 \right| + {\rho _{01}}(t)\\ \otimes \left| 0 \right\rangle \left\langle 1 \right| + {\rho _{10}}(t) \otimes \left| 1 \right\rangle \left\langle 0 \right| + {\rho _{11}}(t)\\ \otimes \left| 1 \right\rangle \left\langle 1 \right|\end{array}\]](https://www.georgeshiber.com/wp-content/ql-cache/quicklatex.com-be477481984ca97258ff3dc5d8e86626_l3.png)
So the master equation reduces component-wise to:
![Rendered by QuickLaTeX.com \[\begin{array}{c}{{\dot \rho }_{00}} = - \left[ {\hat H,{\rho _{00}}} \right] - i\kappa {{\hat a}^\dagger }{\rho _{10}} + i\kappa {\rho _{01}}\hat a\\ + {\Gamma _1}{\rho _{11}}\end{array}\]](https://www.georgeshiber.com/wp-content/ql-cache/quicklatex.com-4584090feb53132b2812d5ca794d18c3_l3.png)
![Rendered by QuickLaTeX.com \[\begin{array}{l}{{\dot \rho }_{01}} = - \left[ {\hat H,{\rho _{01}}} \right] - i\kappa {{\hat a}^\dagger }{\rho _{11}} + i\kappa {\rho _{00}}{{\hat a}^\dagger }\\ - G{\rho _{01}} = \dot \rho _{10}^\dagger \end{array}\]](https://www.georgeshiber.com/wp-content/ql-cache/quicklatex.com-3c059562941350d2d03f665e4852a4c2_l3.png)
and
![Rendered by QuickLaTeX.com \[\begin{array}{c}{{\dot \rho }_{11}} = - \left[ {\hat H,{\rho _{11}}} \right] - i\kappa \hat a{\rho _{10}} + i\kappa {\rho _{10}}{{\hat a}^\dagger }\\ - {\Gamma _1}{\rho _{11}}\end{array}\]](https://www.georgeshiber.com/wp-content/ql-cache/quicklatex.com-d140d6bd4fa2fb8a99347ba92376b33d_l3.png)
with:
![]()
Now, given that the ![]() components are heavily damped, one can adiabatically eliminate all except
components are heavily damped, one can adiabatically eliminate all except ![]() :
:
![Rendered by QuickLaTeX.com \[\begin{array}{l}{{\dot \rho }_{00}} = - i\left[ {{{\hat H}_0},{\rho _{00}}} \right] + \frac{{2{\kappa ^2}}}{G}\hat a{\rho _{00}}{{\hat a}^\dagger }\\ - \frac{{{\kappa ^2}}}{G}{{\hat a}^\dagger }\hat a{\rho _{00}} - \frac{{{\kappa ^2}}}{G}{\rho _{00}}{{\hat a}^\dagger }\hat a\end{array}\]](https://www.georgeshiber.com/wp-content/ql-cache/quicklatex.com-2592efca0fac269fba36c3a766a73687_l3.png)
Such a master equation can be fleshed out as a sum over quantum jump trajectories. So, let us define a non-Hermitian effective Hamiltonian:
![]()
Hence, ![]() evolves according to the Schrödinger equation:
evolves according to the Schrödinger equation:
![Rendered by QuickLaTeX.com \[\frac{{d\left| {\psi _t^{S,m,c}} \right\rangle }}{{dt}} = - \frac{i}{\hbar }{\hat H_{eff}}\left| {\psi _t^{S,m,c}} \right\rangle \]](https://www.georgeshiber.com/wp-content/ql-cache/quicklatex.com-dc22994ac2ae41410b54489844bc5702_l3.png)
with interruptions at random times by sudden quantum jumps:
![]()
that correspond to informational detection of photons.
and this is key: the evolution does not preserve the norm of the state. The physical state is 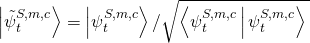 , the renormalized state
, the renormalized state
It follows then that the probability that any given initial state ![]() evolves for a time
evolves for a time ![]() that undergoes
that undergoes ![]() jumps during intervals
jumps during intervals ![]() centered at times
centered at times ![]() is given by:
is given by:
![Rendered by QuickLaTeX.com \[\begin{array}{l}{\left( {2\delta t{\kappa ^2}/G} \right)^N}{\rm{Tr}}\left\{ {{e^{ - i{{\tilde H}_{eff}}\left( {T - {t_N}} \right)}}} \right. \cdot \\\hat a{e^{ - i{{\hat H}_{eff}}}}\left( {{t_N} - {t_{N - 1}}} \right)\hat a...\,\hat a{e^{ - i{{\hat H}_{eff}}t}}\\ \times \left| {\psi _t^{S,m,c}} \right\rangle \left\langle {\psi _t^{S,m,c}} \right|{e^{i{{\tilde H}^\dagger }_{eff}{t_1}}}{{\hat a}^\dagger }...\,\left. {{{\hat a}^\dagger }{e^{i{{\tilde H}^\dagger }_{eff}\left( {T - {t_N}} \right)}}} \right\}\end{array}\]](https://www.georgeshiber.com/wp-content/ql-cache/quicklatex.com-3f372d7bd33398bf84d97f2a72a11170_l3.png)
So, the master equation:
![Rendered by QuickLaTeX.com \[\begin{array}{l}{{\dot \rho }_{00}} = - i\left[ {{{\hat H}_0},{\rho _{00}}} \right] + \frac{{2{\kappa ^2}}}{G}\hat a{\rho _{00}}{{\hat a}^\dagger }\\ - \frac{{{\kappa ^2}}}{G}{{\hat a}^\dagger }\hat a{\rho _{00}} - \frac{{{\kappa ^2}}}{G}{\rho _{00}}{{\hat a}^\dagger }\hat a\end{array}\]](https://www.georgeshiber.com/wp-content/ql-cache/quicklatex.com-2592efca0fac269fba36c3a766a73687_l3.png)
is valid iff the Markovian approximation is faithful and valid only on time-scales longer than ![]() , hence the jump occurs during an interval
, hence the jump occurs during an interval ![]() centered on
centered on ![]() .
.
Now, by taking the average of ![]() over all possible trajectories with the probability measure:
over all possible trajectories with the probability measure:
![Rendered by QuickLaTeX.com \[\begin{array}{l}{\left( {2\delta t{\kappa ^2}/G} \right)^N}{\rm{Tr}}\left\{ {{e^{ - i{{\tilde H}_{eff}}\left( {T - {t_N}} \right)}}} \right. \cdot \\\hat a{e^{ - i{{\hat H}_{eff}}}}\left( {{t_N} - {t_{N - 1}}} \right)\hat a...\,\hat a{e^{ - i{{\hat H}_{eff}}t}}\\ \times \left| {\psi _t^{S,m,c}} \right\rangle \left\langle {\psi _t^{S,m,c}} \right|{e^{i{{\tilde H}^\dagger }_{eff}{t_1}}}{{\hat a}^\dagger }...\,\left. {{{\hat a}^\dagger }{e^{i{{\tilde H}^\dagger }_{eff}\left( {T - {t_N}} \right)}}} \right\}\end{array}\]](https://www.georgeshiber.com/wp-content/ql-cache/quicklatex.com-3f372d7bd33398bf84d97f2a72a11170_l3.png)
the unraveling reproduces the master equation:
![Rendered by QuickLaTeX.com \[\begin{array}{l}{{\dot \rho }_{00}} = - i\left[ {{{\hat H}_0},{\rho _{00}}} \right] + \frac{{2{\kappa ^2}}}{G}\hat a{\rho _{00}}{{\hat a}^\dagger }\\ - \frac{{{\kappa ^2}}}{G}{{\hat a}^\dagger }\hat a{\rho _{00}} - \frac{{{\kappa ^2}}}{G}{\rho _{00}}{{\hat a}^\dagger }\hat a\end{array}\]](https://www.georgeshiber.com/wp-content/ql-cache/quicklatex.com-2592efca0fac269fba36c3a766a73687_l3.png)
let us consider now the decoherent histories picture
A set of decoherent histories for a system is given by choosing a complete set of projections:
![]()
at a sequence of times ![]() representing exclusively different alternatives histories:
representing exclusively different alternatives histories:
![Rendered by QuickLaTeX.com \[\left\{ {\begin{array}{*{20}{c}}{\sum\limits_{{\alpha _j}} {\hat P_{{\alpha _j}}^j\left( {{t_j}} \right) = \hat 1} }\\{\hat P_{{\alpha _j}}^j\left( {{t_j}} \right)\hat P_{{{\alpha '}_j}}^j\left( {{t_j}} \right) = {\delta _{{\alpha _j}{{\alpha '}_j}}}\hat P_{{\alpha _j}}^j\left( {{t_j}} \right)}\end{array}} \right.\]](https://www.georgeshiber.com/wp-content/ql-cache/quicklatex.com-9334403cd84861c67991da11df12e8b6_l3.png)
A history ![]() is given by picking one
is given by picking one ![]() at each point in time. Then the decoherence functional for a given pair of histories
at each point in time. Then the decoherence functional for a given pair of histories ![]() and
and ![]() is:
is:
![Rendered by QuickLaTeX.com \[\begin{array}{l}{D^{dec}}\left[ {h,h'} \right] = {\rm{Tr}}\left\{ {\hat P_{{\alpha _N}}^N\left( {{t_N}} \right)...\hat P_{{\alpha _1}}^1} \right.\left( {{t_1}} \right)\\\rho \left( {{t_0}} \right)\hat P_{{{\alpha '}_1}}^1\left( {{t_1}} \right)...\left. {\hat P_{{{\alpha '}_N}}^N} \right\}\end{array}\]](https://www.georgeshiber.com/wp-content/ql-cache/quicklatex.com-72cb63b6a929efc3e09ad4bd7fc1ea62_l3.png)
and satisfies the decoherence criterion iff the off-diagonal terms vanish, that is:
![]()
Now take any initial pure state:
![]()
and take histories composed only of the Schrödinger-projections:
![]()
characterizing the absence or presence of an m-photon in the output mode. Schrödinger-projections are spaced ![]() -apart, and a history consists of
-apart, and a history consists of ![]() projections representing a total time
projections representing a total time ![]() . Using the quantum regression theorem, the above decoherence functional reduces to:
. Using the quantum regression theorem, the above decoherence functional reduces to:
![Rendered by QuickLaTeX.com \[\begin{array}{l}{D^{dec}}\left[ {h,h'} \right] = {\rm{Tr}}\left\{ {{{\hat P}_{{\alpha _N}}}} \right.{e^{L_s^L\delta t}}\left( {{{\hat P}_{{\alpha _{N - 1}}}}{e^{L_s^L\delta t}}} \right.\\\left( {...} \right.{e^{L_s^L\delta t}}\left( {{{\hat P}_{{\alpha _1}}}} \right.\left| \Psi \right\rangle \left\langle \Psi \right.\left| {\left. {{{\hat P}_{{\alpha _1}}}} \right)} \right.\left. {...} \right)\left. {{{\hat P}_{{\alpha _N}}}} \right\}\end{array}\]](https://www.georgeshiber.com/wp-content/ql-cache/quicklatex.com-ee8068d65875188cfbacc90b0f8c918a_l3.png)
and the Liouville time evolution superoperators:
![]()
transform pure states into mixed states.
So, from:
![Rendered by QuickLaTeX.com \[\begin{array}{c}{{\dot \rho }_{00}} = - \left[ {\hat H,{\rho _{00}}} \right] - i\kappa {{\hat a}^\dagger }{\rho _{10}} + i\kappa {\rho _{01}}\hat a\\ + {\Gamma _1}{\rho _{11}}\end{array}\]](https://www.georgeshiber.com/wp-content/ql-cache/quicklatex.com-4584090feb53132b2812d5ca794d18c3_l3.png)
![Rendered by QuickLaTeX.com \[\begin{array}{l}{{\dot \rho }_{01}} = - \left[ {\hat H,{\rho _{01}}} \right] - i\kappa {{\hat a}^\dagger }{\rho _{11}} + i\kappa {\rho _{00}}{{\hat a}^\dagger }\\ - G{\rho _{01}} = \dot \rho _{10}^\dagger \end{array}\]](https://www.georgeshiber.com/wp-content/ql-cache/quicklatex.com-3c059562941350d2d03f665e4852a4c2_l3.png)
and
![Rendered by QuickLaTeX.com \[\begin{array}{c}{{\dot \rho }_{11}} = - \left[ {\hat H,{\rho _{11}}} \right] - i\kappa \hat a{\rho _{10}} + i\kappa {\rho _{10}}{{\hat a}^\dagger }\\ - {\Gamma _1}{\rho _{11}}\end{array}\]](https://www.georgeshiber.com/wp-content/ql-cache/quicklatex.com-d140d6bd4fa2fb8a99347ba92376b33d_l3.png)
one can uniquely determine the character of the different histories, and the central regime is in the range:
![]()
Note, the ![]() terms on this time-scale are sufficient to guarantee decoherence and what is crucial is that the
terms on this time-scale are sufficient to guarantee decoherence and what is crucial is that the ![]() terms resolve into individual pure state trajectories: and that is because
terms resolve into individual pure state trajectories: and that is because
the probability of a photon being emitted in any single time-step is small yet any emission implies there is a non-zero probability of absorption on a time scale 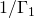
The decoherence-effect effectively gives rise to the terms:
![]()
in equation:
![Rendered by QuickLaTeX.com \[\begin{array}{l}{{\dot \rho }_{00}} = - i\left[ {{{\hat H}_0},{\rho _{00}}} \right] + \frac{{2{\kappa ^2}}}{G}\hat a{\rho _{00}}{{\hat a}^\dagger }\\ - \frac{{{\kappa ^2}}}{G}{{\hat a}^\dagger }\hat a{\rho _{00}} - \frac{{{\kappa ^2}}}{G}{\rho _{00}}{{\hat a}^\dagger }\hat a\end{array}\]](https://www.georgeshiber.com/wp-content/ql-cache/quicklatex.com-2592efca0fac269fba36c3a766a73687_l3.png)
and are factored in the effective Hamiltonian:
![]()
and such terms are fundamental on a time-scale ![]() , unlike
, unlike ![]() , which becomes fundamental on a time scale
, which becomes fundamental on a time scale ![]()
It is the term:
![]()
that causes pure states to evolve into mixed states in:
![Rendered by QuickLaTeX.com \[\begin{array}{l}{{\dot \rho }_{00}} = - i\left[ {{{\hat H}_0},{\rho _{00}}} \right] + \frac{{2{\kappa ^2}}}{G}\hat a{\rho _{00}}{{\hat a}^\dagger }\\ - \frac{{{\kappa ^2}}}{G}{{\hat a}^\dagger }\hat a{\rho _{00}} - \frac{{{\kappa ^2}}}{G}{\rho _{00}}{{\hat a}^\dagger }\hat a\end{array}\]](https://www.georgeshiber.com/wp-content/ql-cache/quicklatex.com-2592efca0fac269fba36c3a766a73687_l3.png)
One can maintain the purity of the system-state over a full trajectory by picking a time: ![]() .
.
Hence, with:
![]()
and after evolving for a time ![]() , the state becomes:
, the state becomes:
![Rendered by QuickLaTeX.com \[\begin{array}{*{20}{l}}{{{\left( {{e^{L_s^L\delta t}}\rho } \right)}_{00}} = {\rho _{00}} - i\left[ {{{\hat H}_0},{\rho _{00}}} \right]\delta t}\\{ - \frac{{{\kappa ^2}}}{G}{{\hat a}^\dagger }\hat a{\rho _{00}}\delta t - \frac{{{\kappa ^2}}}{G}{\rho _{00}}{{\hat a}^\dagger }\hat a\delta t + {\rm{h}}.{\rm{o}}.{\rm{t}}}\\{ \approx {e^{ - i\left( {{{\hat H}_0} - i\left( {{\kappa ^2}/G} \right){{\hat a}^\dagger }\hat a} \right)\delta t}}{\rho _{00}}{e^{i\left( {{{\hat H}_0} + i\left( {{\kappa ^2}/G} \right){{\hat a}^\dagger }\hat a} \right)\delta t}}}\end{array}\]](https://www.georgeshiber.com/wp-content/ql-cache/quicklatex.com-0024936fc607d64f39314d08ab2a7a40_l3.png)
![Rendered by QuickLaTeX.com \[\begin{array}{l}{\left( {{e^{L_s^L\delta t}}\rho } \right)_{01}} = \frac{{i\kappa }}{G}{\rho _{00}}{{\hat a}^\dagger } + {\rm{h}}{\rm{.o}}{\rm{.t}}{\rm{.}} = \\\left( {{e^{L_s^L\delta t}}\rho } \right)_{10}^\dagger \end{array}\]](https://www.georgeshiber.com/wp-content/ql-cache/quicklatex.com-b8714dcb786a97d04c599f6028e9eeb7_l3.png)
and
![]()
Thus, the effective Hamiltonian again has two crucial properties. One is the possibility that the excited-mode photon will be absorbed by the measuring device. The second, negligible though, is the possibility that the excited-mode photon will be coherently re-absorbed by the measuring system.
Now, by combining the above three expressions with the projections:
![]()
we can derive the probabilities for all possible histories. Take the history given by an unbroken string of ![]()
![]() projections, corresponding to no excited-mode photon being emitted during a time
projections, corresponding to no excited-mode photon being emitted during a time ![]() .
.
The probability of such a history is the diagonal element of:
![Rendered by QuickLaTeX.com \[\begin{array}{l}{D^{dec}}\left[ {h,h'} \right] = {\rm{Tr}}\left\{ {{{\hat P}_{{\alpha _N}}}} \right.{e^{L_s^L\delta t}}\left( {{{\hat P}_{{\alpha _{N - 1}}}}{e^{L_s^L\delta t}}} \right.\\\left( {...} \right.{e^{L_s^L\delta t}}\left( {{{\hat P}_{{\alpha _1}}}} \right.\left| \Psi \right\rangle \left\langle \Psi \right.\left| {\left. {{{\hat P}_{{\alpha _1}}}} \right)} \right.\left. {...} \right)\left. {{{\hat P}_{{\alpha _N}}}} \right\}\end{array}\]](https://www.georgeshiber.com/wp-content/ql-cache/quicklatex.com-ee8068d65875188cfbacc90b0f8c918a_l3.png)
Now, expanding the time evolution superoperator using:
![Rendered by QuickLaTeX.com \[\begin{array}{*{20}{l}}{{{\left( {{e^{L_s^L\delta t}}\rho } \right)}_{00}} = {\rho _{00}} - i\left[ {{{\hat H}_0},{\rho _{00}}} \right]\delta t}\\{ - \frac{{{\kappa ^2}}}{G}{{\hat a}^\dagger }\hat a{\rho _{00}}\delta t - \frac{{{\kappa ^2}}}{G}{\rho _{00}}{{\hat a}^\dagger }\hat a\delta t + {\rm{h}}.{\rm{o}}.{\rm{t}}}\\{ \approx {e^{ - i\left( {{{\hat H}_0} - i\left( {{\kappa ^2}/G} \right){{\hat a}^\dagger }\hat a} \right)\delta t}}{\rho _{00}}{e^{i\left( {{{\hat H}_0} + i\left( {{\kappa ^2}/G} \right){{\hat a}^\dagger }\hat a} \right)\delta t}}}\end{array}\]](https://www.georgeshiber.com/wp-content/ql-cache/quicklatex.com-0024936fc607d64f39314d08ab2a7a40_l3.png)
![Rendered by QuickLaTeX.com \[\begin{array}{l}{\left( {{e^{L_s^L\delta t}}\rho } \right)_{01}} = \frac{{i\kappa }}{G}{\rho _{00}}{{\hat a}^\dagger } + {\rm{h}}{\rm{.o}}{\rm{.t}}{\rm{.}} = \\\left( {{e^{L_s^L\delta t}}\rho } \right)_{10}^\dagger \end{array}\]](https://www.georgeshiber.com/wp-content/ql-cache/quicklatex.com-b8714dcb786a97d04c599f6028e9eeb7_l3.png)
and
![]()
one can see now that after ![]() we get:
we get:
![Rendered by QuickLaTeX.com \[\begin{array}{l}{{\hat P}_0}{e^{L_s^L}}\left( {\left| \psi \right\rangle \left\langle \psi \right| \otimes \left| 0 \right\rangle \left\langle 0 \right|} \right){{\hat P}_0} \approx \\\left( {{e^{ - i\left( {{{\hat H}_0} - i\left( {{\kappa ^2}/G} \right){{\hat a}^\dagger }\hat a} \right)\delta t}}\left| \psi \right\rangle \left\langle \psi \right|{e^{i\left( {{{\hat H}_0} + i\left( {{\kappa ^2}/G} \right){{\hat a}^\dagger }\hat a} \right)\delta t}}} \right)\left| 0 \right\rangle \left\langle 0 \right|\end{array}\]](https://www.georgeshiber.com/wp-content/ql-cache/quicklatex.com-112c046e7b6166d5c4f16f1a37214d3b_l3.png)
Taking the trace after ![]() repetitions, one gets:
repetitions, one gets:
![]()
which is the probability of the quantum jump trajectory when no jumps are detected
Now, let a photon be emitted at time ![]() and with projection
and with projection ![]() instead. The corresponding probability is then:
instead. The corresponding probability is then:
![Rendered by QuickLaTeX.com \[\begin{array}{l}p(h) \approx \left( {2\delta t{\kappa ^2}/G} \right)\\{\rm{Tr}}\left\{ {\hat a{e^{ - i{{\hat H}_{eff}}N\delta t}}\left| \psi \right\rangle \left\langle \psi \right|{e^{i{{\hat H}^\dagger }_{eff}N\delta t}}{{\hat a}^\dagger }} \right\}\end{array}\]](https://www.georgeshiber.com/wp-content/ql-cache/quicklatex.com-d409bd925d134b61a2d5d39960859023_l3.png)
again, this is the probability of the corresponding quantum jump trajectory
The question then is: what occurs after the output mode has registered as being in the excited state? Two possibilities exist. One is that the output mode drops back to the un-excited state: measuring state absorption, represented by:
![]()
Or a constant exited state:
![Rendered by QuickLaTeX.com \[\begin{array}{l}{{\hat P}_1}{e^{L_s^L\delta t}}\left( {\left| {\psi '} \right\rangle \left\langle {\psi '} \right| \otimes \left| 1 \right\rangle \left\langle 1 \right|} \right){{\hat P}_1} \approx \\\left( {1 - {\Gamma _1}\delta t} \right){e^{ - i{{\hat H}_{eff}}\delta t}}\left| {\psi '} \right\rangle \left\langle {\psi '} \right|\\{e^{i{{\hat H}^\dagger }_{eff}\delta t}} \otimes \left| 1 \right\rangle \left\langle 1 \right|\end{array}\]](https://www.georgeshiber.com/wp-content/ql-cache/quicklatex.com-db07047f772c6c0c456047c5effaed6a_l3.png)
So, the output mode has probability ![]()
![]() per
per ![]() of regressing back to the ground state, while the system state continues to evolve in accordance with the effective Hamiltonian
of regressing back to the ground state, while the system state continues to evolve in accordance with the effective Hamiltonian ![]() .
.
Hence, we have a near-unity probability of the external mode returning to the ground state within a time of order 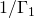 , thus we can sum over all histories in which the photon is absorbed within this time and again this matches the quantum jump trajectories exactly
, thus we can sum over all histories in which the photon is absorbed within this time and again this matches the quantum jump trajectories exactly
Generalizing, the probability of such histories will be exactly of the form:
![Rendered by QuickLaTeX.com \[\begin{array}{l}{\left( {2\delta t{\kappa ^2}/G} \right)^N}{\rm{Tr}}\left\{ {{e^{ - i{{\tilde H}_{eff}}\left( {T - {t_N}} \right)}}} \right. \cdot \\\hat a{e^{ - i{{\hat H}_{eff}}}}\left( {{t_N} - {t_{N - 1}}} \right)\hat a...\,\hat a{e^{ - i{{\hat H}_{eff}}t}}\\ \times \left| {\psi _t^{S,m,c}} \right\rangle \left\langle {\psi _t^{S,m,c}} \right|{e^{i{{\tilde H}^\dagger }_{eff}{t_1}}}{{\hat a}^\dagger }...\,\left. {{{\hat a}^\dagger }{e^{i{{\tilde H}^\dagger }_{eff}\left( {T - {t_N}} \right)}}} \right\}\end{array}\]](https://www.georgeshiber.com/wp-content/ql-cache/quicklatex.com-3f372d7bd33398bf84d97f2a72a11170_l3.png)
To decohere histories, the following must hold, for ![]() :
:
![]()
for each separate ![]() ,
, ![]() . Hence, it suffices to analyze two histories which differ at a single time
. Hence, it suffices to analyze two histories which differ at a single time ![]() , one with projection
, one with projection ![]() , the other
, the other ![]() ,
,
which is equivalent to picking out the 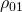 and
and 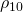 component of
component of 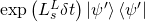 at that time
at that time
arriving at:
![Rendered by QuickLaTeX.com \[\frac{{{{\left| {\tilde D\left[ {h,h'} \right]} \right|}^2}}}{{p(h)p(h')}} \sim \frac{1}{{{{\left( {G\delta t} \right)}^2}}}\]](https://www.georgeshiber.com/wp-content/ql-cache/quicklatex.com-f09562c6431a652bb35cf572ae7b77c4_l3.png)
with the sum rules given by ![]() .
.
Concluding then, we have demonstrated that continuous measurements are given by the set of quantum jump trajectories corresponding to a set of decoherent histories that reproduces quantum mechanics as a Bayesian theory over Hilbert space with complex ring.


1 Response
The Cosmological Quantum State from Deformation Quantization
Saturday, December 24, 2016[…] as I showed: the Heisenberg equation with respect to the Lindblad collapse operators {ell _{k,t}}: […]